The process has been shown to be impossible as described. Suppose we want to make some modification to make the process possible such that it can deliver the promised 2000 kJ of heat.
Hint: Because the solution involves some repetition, this problem would be easier to do if you do the calculation on a spreadsheet or a Matlab script.
a) Determine the temperature of the hot reservoir that would make the process possible, keeping everything else the same. Is this reservoir temperature higher or lower than the reservoir temperature in the impossible process (200C)? Why?
b) Determine the temperature of inlet saturated steam that would make the process possible, keeping everything else the same. Is this steam temperature higher or lower than the steam temperature in the impossible process (100C)? Why?
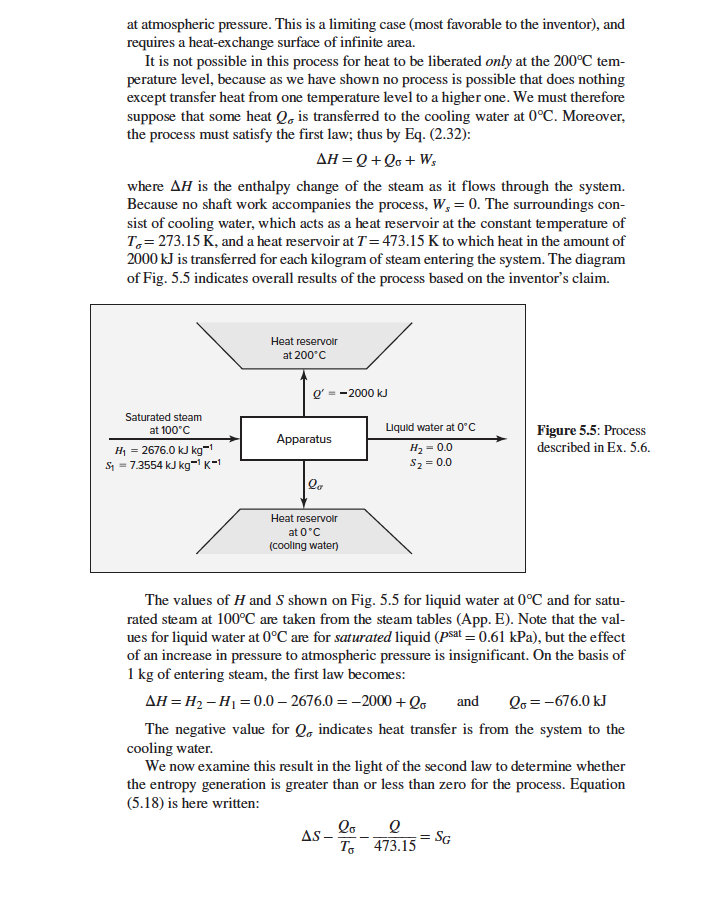

Example 5.6 An inventor claims to have devised a process which takes in only saturated steam at 100C and which by a complicated series of steps makes heat continuously available at a temperature level of 200C, with 2000 kJ of energy available at 200C for every kilogram of steam taken into the process. Show whether or not this process is possible. To give this process the most favorable conditions, assume cooling water available in unlimited quantity at a temperature of 0C. Solution 5.6 For any process to be even theoretically possible, it must meet the requirements of the first and second laws of thermodynamics. The detailed mechanism need not be known to determine whether this is true; only the overall result is required. If the claims of the inventor satisfy the laws of thermodynamics, fulfilling the claims is theoretically possible. The determination of a mechanism is then a matter of ingenuity. Otherwise, the process is impossible, and no mechanism for carrying it out can be devised. In the present instance, a continuous process takes in saturated steam at 100C, and makes heat Q continuously available at a temperature level of 200C. Because cooling water is available at 0C, the maximum possible use is made of the steam if it is condensed and cooled (without freezing) to this exit temperature and discharged at atmospheric pressure. This is a limiting case (most favorable to the inventor), and requires a heat-exchange surface of infinite area. It is not possible in this process for heat to be liberated only at the 200C tem- perature level, because as we have shown no process is possible that does nothing except transfer heat from one temperature level to a higher one. We must therefore suppose that some heat Qo is transferred to the cooling water at 0C. Moreover, the process must satisfy the first law; thus by Eq. (2.32): AH = Q + Qo+W, where AH is the enthalpy change of the steam as it flows through the system. Because no shaft work accompanies the process, W, = 0. The surroundings con- sist of cooling water, which acts as a heat reservoir at the constant temperature of To= 273.15 K, and a heat reservoir at T=473.15 K to which heat in the amount of 2000 kJ is transferred for each kilogram of steam entering the system. The diagram of Fig. 5.5 indicates overall results of the process based on the inventor's claim. Heat reservoir at 200C Q = -2000 kJ Saturated steam at 100C Hy = 2676.0 kJ kg- S = 7.3554 kJ kg-K- Apparatus Liquid water at 0C H2 = 0.0 $z=0.0 Figure 5.5: Process described in Ex. 5.6. = Q Heat reservoir at 0C (cooling water) and The values of H and S shown on Fig. 5.5 for liquid water at 0C and for satu- rated steam at 100C are taken from the steam tables (App. E). Note that the val- ues for liquid water at 0C are for saturated liquid (psat = 0.61 kPa), but the effect of an increase in pressure to atmospheric pressure is insignificant. On the basis of 1 kg of entering steam, the first law becomes: AH = H2 - H = 0.0 - 2676.0 = -2000 + lo Qo=-676.0 kJ The negative value for Q. indicates heat transfer is from the system to the cooling water. We now examine this result in the light of the second law to determine whether the entropy generation is greater than or less than zero for the process. Equation (5.18) is here written: Q lo AS- To 473.15 =SG = For 1 kg of steam, AS=S2-S = 0.0000 - 7.3554= -7.3554 kJ.K-1 The entropy generation is then: -676.0 -2000 SG=-7.3554 - 273.15 473.15 =-7.3554 +4.2270 +2.4748= -0.6536 kJ-K-1 This negative result means that the process as described is impossible; the second law in the form of Eq. (5.18) requires SG 20. The negative result of the preceding example does not mean that all processes of this general nature are impossible; only that the inventor claimed too much. Indeed, the maximum amount of heat which can be transferred to the hot reservoir at 200C is readily calculated. The energy balance is: Q+Qo = AH (A) The maximum heat transfer to the hot reservoir occurs when the process is completely revers- ible, in which case SG=0, and Eq. (5.18) becomes e lo = AS (B) T'To Combination of Eqs. (A) and (B) and solution for Qyields: - ( - ..S) Q=T-To With respect to the preceding example, 473.15 -2676.0 (273.15) 7.3554)] Q = = - 1577.7 kJ kg- 200 This value is smaller in magnitude than the 2000 kJ-kg-1 claimed. Example 5.6 An inventor claims to have devised a process which takes in only saturated steam at 100C and which by a complicated series of steps makes heat continuously available at a temperature level of 200C, with 2000 kJ of energy available at 200C for every kilogram of steam taken into the process. Show whether or not this process is possible. To give this process the most favorable conditions, assume cooling water available in unlimited quantity at a temperature of 0C. Solution 5.6 For any process to be even theoretically possible, it must meet the requirements of the first and second laws of thermodynamics. The detailed mechanism need not be known to determine whether this is true; only the overall result is required. If the claims of the inventor satisfy the laws of thermodynamics, fulfilling the claims is theoretically possible. The determination of a mechanism is then a matter of ingenuity. Otherwise, the process is impossible, and no mechanism for carrying it out can be devised. In the present instance, a continuous process takes in saturated steam at 100C, and makes heat Q continuously available at a temperature level of 200C. Because cooling water is available at 0C, the maximum possible use is made of the steam if it is condensed and cooled (without freezing) to this exit temperature and discharged at atmospheric pressure. This is a limiting case (most favorable to the inventor), and requires a heat-exchange surface of infinite area. It is not possible in this process for heat to be liberated only at the 200C tem- perature level, because as we have shown no process is possible that does nothing except transfer heat from one temperature level to a higher one. We must therefore suppose that some heat Qo is transferred to the cooling water at 0C. Moreover, the process must satisfy the first law; thus by Eq. (2.32): AH = Q + Qo+W, where AH is the enthalpy change of the steam as it flows through the system. Because no shaft work accompanies the process, W, = 0. The surroundings con- sist of cooling water, which acts as a heat reservoir at the constant temperature of To= 273.15 K, and a heat reservoir at T=473.15 K to which heat in the amount of 2000 kJ is transferred for each kilogram of steam entering the system. The diagram of Fig. 5.5 indicates overall results of the process based on the inventor's claim. Heat reservoir at 200C Q = -2000 kJ Saturated steam at 100C Hy = 2676.0 kJ kg- S = 7.3554 kJ kg-K- Apparatus Liquid water at 0C H2 = 0.0 $z=0.0 Figure 5.5: Process described in Ex. 5.6. = Q Heat reservoir at 0C (cooling water) and The values of H and S shown on Fig. 5.5 for liquid water at 0C and for satu- rated steam at 100C are taken from the steam tables (App. E). Note that the val- ues for liquid water at 0C are for saturated liquid (psat = 0.61 kPa), but the effect of an increase in pressure to atmospheric pressure is insignificant. On the basis of 1 kg of entering steam, the first law becomes: AH = H2 - H = 0.0 - 2676.0 = -2000 + lo Qo=-676.0 kJ The negative value for Q. indicates heat transfer is from the system to the cooling water. We now examine this result in the light of the second law to determine whether the entropy generation is greater than or less than zero for the process. Equation (5.18) is here written: Q lo AS- To 473.15 =SG = For 1 kg of steam, AS=S2-S = 0.0000 - 7.3554= -7.3554 kJ.K-1 The entropy generation is then: -676.0 -2000 SG=-7.3554 - 273.15 473.15 =-7.3554 +4.2270 +2.4748= -0.6536 kJ-K-1 This negative result means that the process as described is impossible; the second law in the form of Eq. (5.18) requires SG 20. The negative result of the preceding example does not mean that all processes of this general nature are impossible; only that the inventor claimed too much. Indeed, the maximum amount of heat which can be transferred to the hot reservoir at 200C is readily calculated. The energy balance is: Q+Qo = AH (A) The maximum heat transfer to the hot reservoir occurs when the process is completely revers- ible, in which case SG=0, and Eq. (5.18) becomes e lo = AS (B) T'To Combination of Eqs. (A) and (B) and solution for Qyields: - ( - ..S) Q=T-To With respect to the preceding example, 473.15 -2676.0 (273.15) 7.3554)] Q = = - 1577.7 kJ kg- 200 This value is smaller in magnitude than the 2000 kJ-kg-1 claimed









