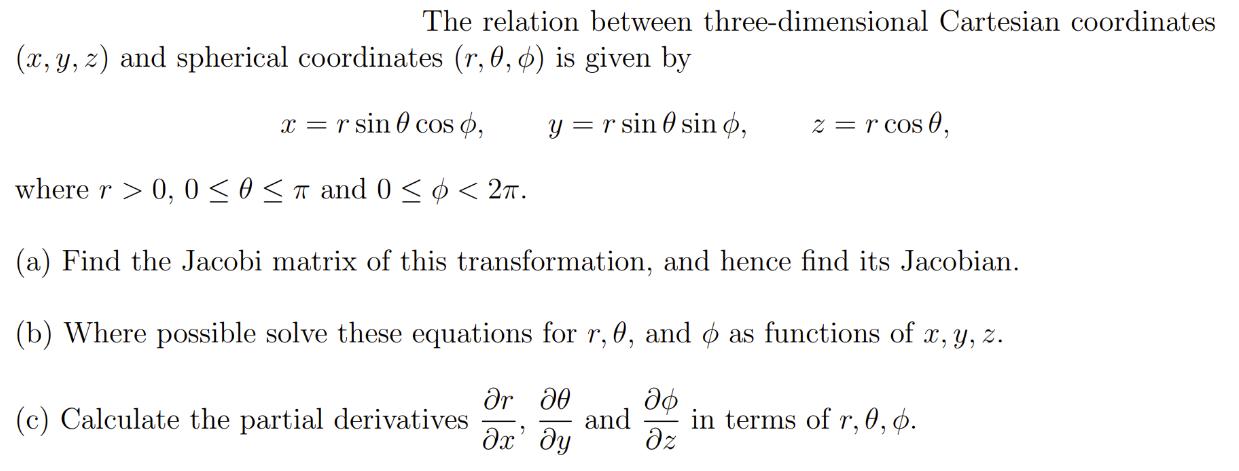
The relation between three-dimensional Cartesian coordinates (x, y, z) and spherical coordinates (r, 0, 0) is given by x = r sin cos, y = r sin 0 sin o, 2 = r cos 0, where > 0, 0 0 and 0 < 2. (a) Find the Jacobi matrix of this transformation, and hence find its Jacobian. (b) Where possible solve these equations for r, 0, and as functions of x, y, z. and (c) Calculate the partial derivatives Jr 20 r in terms of r, 0, p.