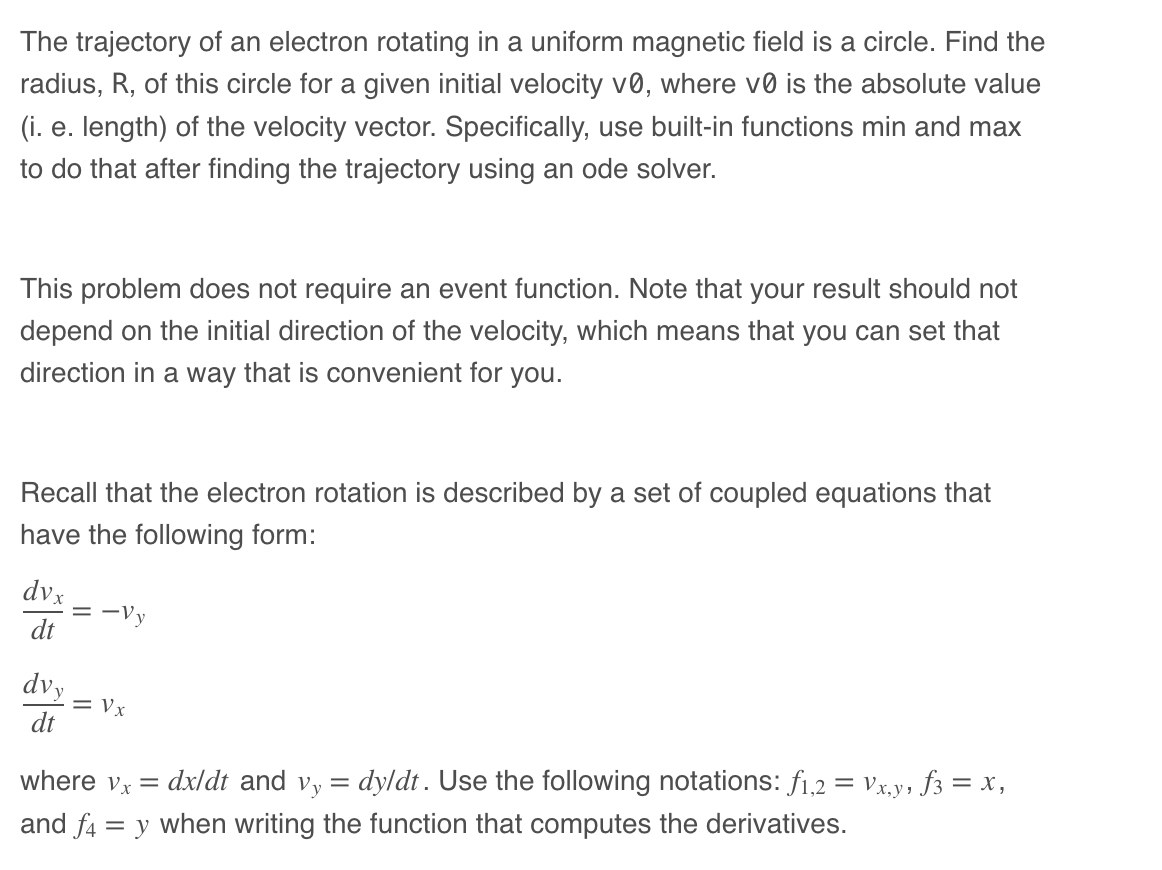
The trajectory of an electron rotating in a uniform magnetic field is a circle. Find the

radius, $R,$ of this circle for a given initial velocity $v0,$ where $v0$ is the absolute value

$($i$.$ e$.$ length$)$ of the velocity vector. Specifically, use built$-$in functions min and max

to do that after finding the trajectory using an ode solver.

This problem does not require an event function. Note that your result should not

depend on the initial direction of the velocity, which means that you can set that

direction in a way that is convenient for you.

Recall that the electron rotation is described by a set of coupled equations that

have the following form:

$\frac{d{v}_x}{dt}=-v_y$

$\frac{d{v}_y}{dt}=v_x$

where $v_x=d\frac{x}{d}t$ and $v_y=d\frac{y}{d}t.$ Use the following notations: $f_{1,2}=v_{x,y},f_3=x,$

and $f_4=y$ when writing the function that computes the derivatives.

This is my code right now but there is an error $($File: solution.m Line: $4$ Column: $30$

Invalid expression. When calling a function or indexing a variable, use parentheses. Otherwise, check for mismatched delimiters.$)$

x$0=0$;

y$0=0$;

v$0=$ randi$(3)$;

$[$t$,$f$]=$ ode$45($@odefun, $[0,10]],[$v$00$ x$0$ y$0])$;

x $=$ f$($:$,3)$;

y $=$ f$($:$,4)$;

plot$($x$,$y$)$

Radius$=$

$\%*********************************$

function dfdt $=$ odefun$($t$,$f$)$

dfdt $=$ zeros$(4,1)$;

dfdt $=$ zeros$(4,1)$;

x $=$ f$(3)$;

y $=$ f$(4)$;

dfdt $=[$y; $-$x; x; y$]$;

end