The uk700 randomized trial [1] compared the 2-year costs (in dollars) of treating mentally-ill patients in the community using two different management approaches: intensive (caseload of 10?15 patients) and standard (caseload of 30?35 patients). Data for 667 patients are available. Numerous models were fitted, including those summarized in For all these models, ?

where x₁ = 1 for the intensive group and is zero otherwise, and x₂ is the patient age in completed years.

?

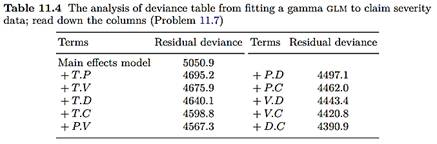1. Based on the aic, which edm seems most appropriate?

2. The constants in the models ?₀ are not revealed. Nonetheless, write down the two models based on this edm as comprehensively as possible.

3. Interpret the regression parameters for x₁ in both models.

4. Interpret the regression parameters for x₂ in both models.

5. Is the type of treatment significant for modelling cost? Explain.

6. Is the patient age significant for modelling cost? Explain.

7. Which interpretation (i.e. the use of which link function) seems most appropriate? Why?

Transcribed Image Text:

g(u) Bo + B121 + ₂x2, g(u) Bo + B121 + ₂x2,

Answer rating: 100% (QA)

1 The AIC is a measure of the goodness of fit of a model The lower the AIC the better the model In t... View the full answer