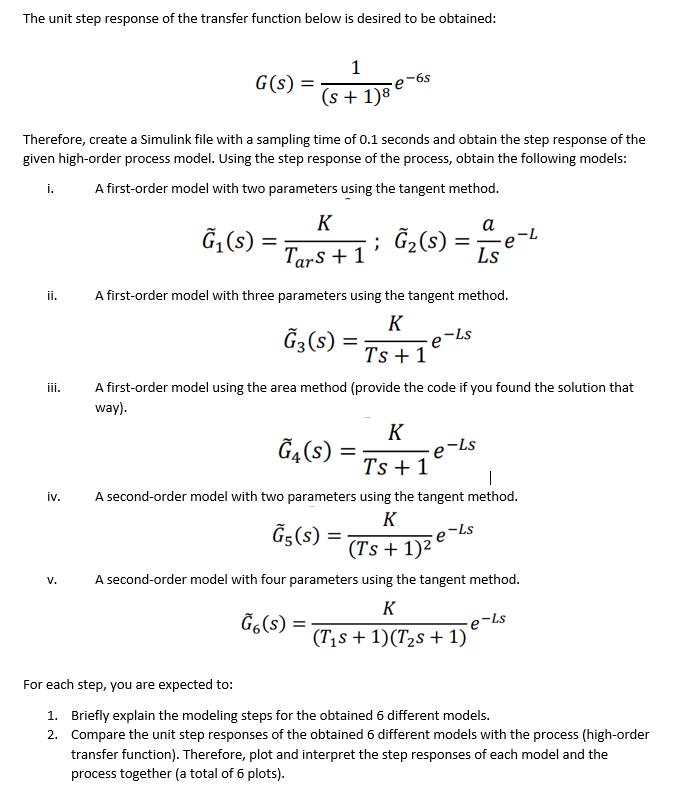
The unit step response of the transfer function below is desired to be obtained: 1 G(s) = -65 (s + 1)8 Therefore, create a Simulink file with a sampling time of 0.1 seconds and obtain the step response of the given high-order process model. Using the step response of the process, obtain the following models: i. A first-order model with two parameters using the tangent method. ii. iii. iv. V. G(s) K a = Tars +1 ; G(s) = e-L Ls A first-order model with three parameters using the tangent method. K G3(S) = -LS Ts + 1 A first-order model using the area method (provide the code if you found the solution that way). K G4(S) e-Ls Ts + 1 A second-order model with two parameters using the tangent method. K G5(s) -Ls (Ts + 1) A second-order model with four parameters using the tangent method. G6(s) K (Ts+1)(T2s+1) e-Ls For each step, you are expected to: 1. Briefly explain the modeling steps for the obtained 6 different models. 2. Compare the unit step responses of the obtained 6 different models with the process (high-order transfer function). Therefore, plot and interpret the step responses of each model and the process together (a total of 6 plots). The unit step response of the transfer function below is desired to be obtained: 1 G(s) = -65 (s + 1)8 Therefore, create a Simulink file with a sampling time of 0.1 seconds and obtain the step response of the given high-order process model. Using the step response of the process, obtain the following models: i. A first-order model with two parameters using the tangent method. ii. iii. iv. V. G(s) K a = Tars +1 ; G(s) = e-L Ls A first-order model with three parameters using the tangent method. K G3(S) = -LS Ts + 1 A first-order model using the area method (provide the code if you found the solution that way). K G4(S) e-Ls Ts + 1 A second-order model with two parameters using the tangent method. K G5(s) -Ls (Ts + 1) A second-order model with four parameters using the tangent method. G6(s) K (Ts+1)(T2s+1) e-Ls For each step, you are expected to: 1. Briefly explain the modeling steps for the obtained 6 different models. 2. Compare the unit step responses of the obtained 6 different models with the process (high-order transfer function). Therefore, plot and interpret the step responses of each model and the process together (a total of 6 plots).