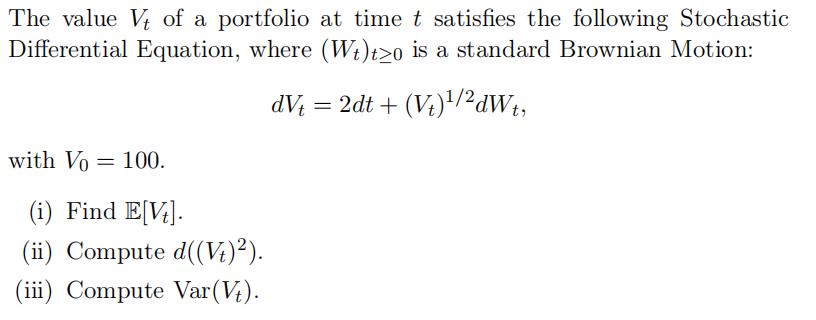
The value Vt of a portfolio at time t satisfies the following Stochastic Differential Equation, where (Wt)tzo is a standard Brownian Motion dV 2dt (V) 2dWt, with Vo 100 (i) Find E V (ii) Compute d((Vt)) (iii) Compute Var(Vt) The value Vt of a portfolio at time t satisfies the following Stochastic ...

i Find EVi EVi ErVidt VidWt EVi rEVidt EVidWt EVi rEVidt ii Compute dV dV 2rVdV dV dV 2rVrVdt ...

The value Vt of a portfolio at time t satisfies the following Stochastic Differential Equation, where...

Fantastic news! We've Found the answer you've been seeking!

Question:

Related Book For answer-question

An Introduction to the Mathematics of Financial Derivatives

ISBN: 978-0123846822

3rd edition

Authors: Ali Hirsa, Salih N. Neftci

See More Books

Posted Date: Oct 12, 2022 05:35 AM

See More Questions

Find an L D VT factorization of the following symmetric matrices. Which are positive definite? a. b. c. d. e. f. g. h. 3 (3 -1) 315 151 313 112 121 211 231 113 2 0112 1011 1201 110 0123 1032 2301...
Find the solution of the differential equation that satisfies the given initial condition. (a) dy/dx = x/y' y(0) = -3 (b) du/dt = 2t + sec2t/2u, u(0) = -5 (c) x 1n x = y(1 + 3 + y2)y', y(1) = -1
Find the solution to the following differential equations: (a) dv / dt + 2v = 0, v(0) = -1v (b) 2 di / dt + 3i = 0, i(0) = 2
George operates a business that generated adjusted gross income of $250,000 and taxable income of $170,000 this year (before the domestic production activities deduction). Included in income was...
Madeline enters into a contract with Canyon Canoes to go on a weeklong canoe trip down a river. The contract states that, although the firm provides experienced guides and high-quality equipment,...
Propose two adjustments that you as a Human Resources manager can make to support the changes related to high-performance work systems, technological changes, and a change in the employment...
Recall, even if evidence is relevant under the general relevance test, there may be special rules that preclude the introduction of the evidence. In addition to a persons character or habits, what...
Recall the Innis Investments problem. Letting S = units purchased in the stock fund M = units purchased in the money market fund Leads to the following formulation: Min8S + 3M s.t. 50S + 100M ...
Describe 3 classes of problems with regard to organizational design that tend to develop in traditionally structured companies that Have grown rapidly with increased staffing complements and new...
Suppose that the point spread for a particular sporting event is 10 points and that with this spread you are convinced you would have a 0.60 probability of winning a bet on your team. However, the...
A copper wire, 3 mm in diameter, is wound about a cylinder whose length is 12 cm, and diameter 10 cm, so as to cover the curved surface of the cylinder. Find the length and mass of the wire, assuming...
Which of the following statements about sequence flows is not true? a. Sequence flows are continuous from the start event through activities and gateways, if any, to an end event in a pool. b....
What is your view on the extent to which accounting practice should be neutral in its treatment of stakeholders, and the extent to which it should be the result of political pressure from those...
Imagine you are planning to invest in a listed company manufacturing high quality footwear. There is a strong family representation on the board of directors and the company has a strong reputation...
How much of the information suggested for the strategic report is extracted directly from the financial statements? How much of the information suggested for the strategic report provides additional...
The SEC requires that, in order to be classified as proved undeveloped reserves, there must be a plan in place to develop those reserves within a specific period of time. That time period is: a....
- What is a scarcity appeal? - Why do scarcity appeals work? - On whom do scarcity appeals work? - What is an example of a scarcity appeal from outside class/the book? Why is it an effective or...
In Problems, solve each system of equations. x + 2y + 3z = 5 y + 11z = 21 5y + 9z = 13
Consider a stock St and a plain vanilla, at-the- money put option written on this stock. The option expires at time t + , where denotes a small interval. At time t, there are only two possible ways...
In Example 7 find the breakeven spread that is the spread that makes present value of the fixed leg the same of present value of the contingent leg.
Show that the price of a swap is the same as the price of a cap minus the price of a floor.
While you're waiting for the ribs to cook, you muse about the propane tank of your barbecue. You're curious about the volume of propane versus the actual tank size. Find the liquid propane volume...
Derive the following conversion factors: (a) Convert a volume flow rate in cubic inches per minute to cubic millimeters per minute. (b) Convert a volume flow rate in cubic meters per second to...
Express the following in $\mathrm{BG}$ units: (a) $50 \mathrm{~m}^{2}$ (b) $250 \mathrm{cc}$ (c) $100 \mathrm{~kW}$ (d) $5 \mathrm{~kg} / \mathrm{m}^{2}$

The value Vt of a portfolio at time t satisfies the following Stochastic Differential Equation, where...

Question:

Expert Answer:

i Find EVi EVi ErVidt VidWt EVi rEVidt EVidWt EVi rEVidt ii Compute dV dV 2rVdV dV dV 2rVrVdt ... View the full answer

An Introduction to the Mathematics of Financial Derivatives

Students also viewed these accounting questions