These two are Applied Math Analysis questions. Please teach me the process detailedly. Thank you so much.

Please help me with both two questions.

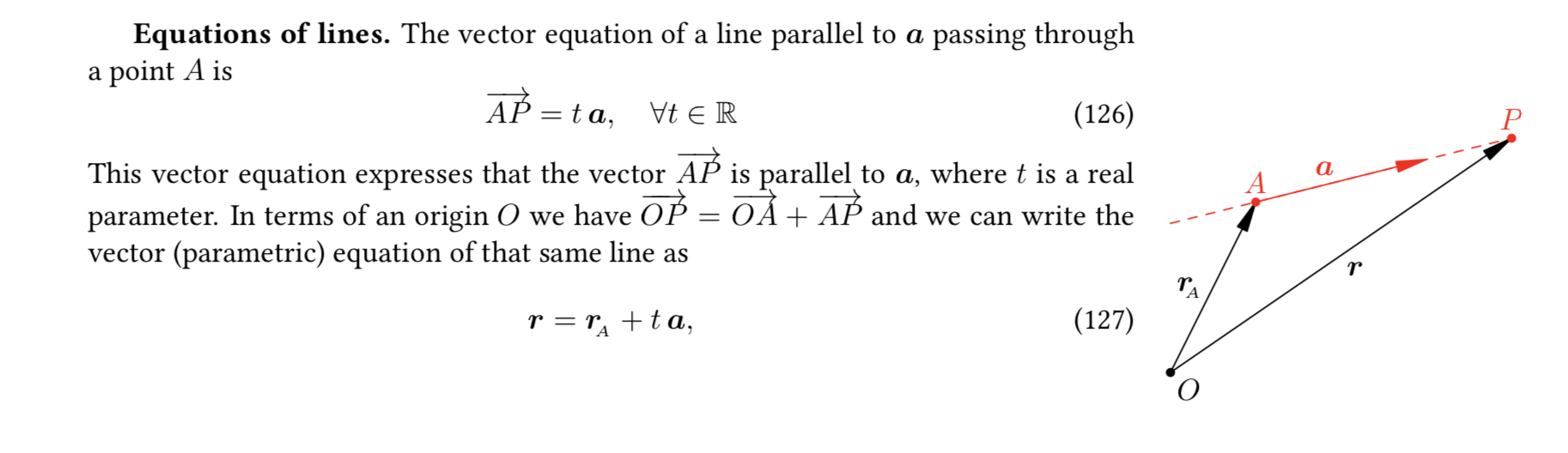
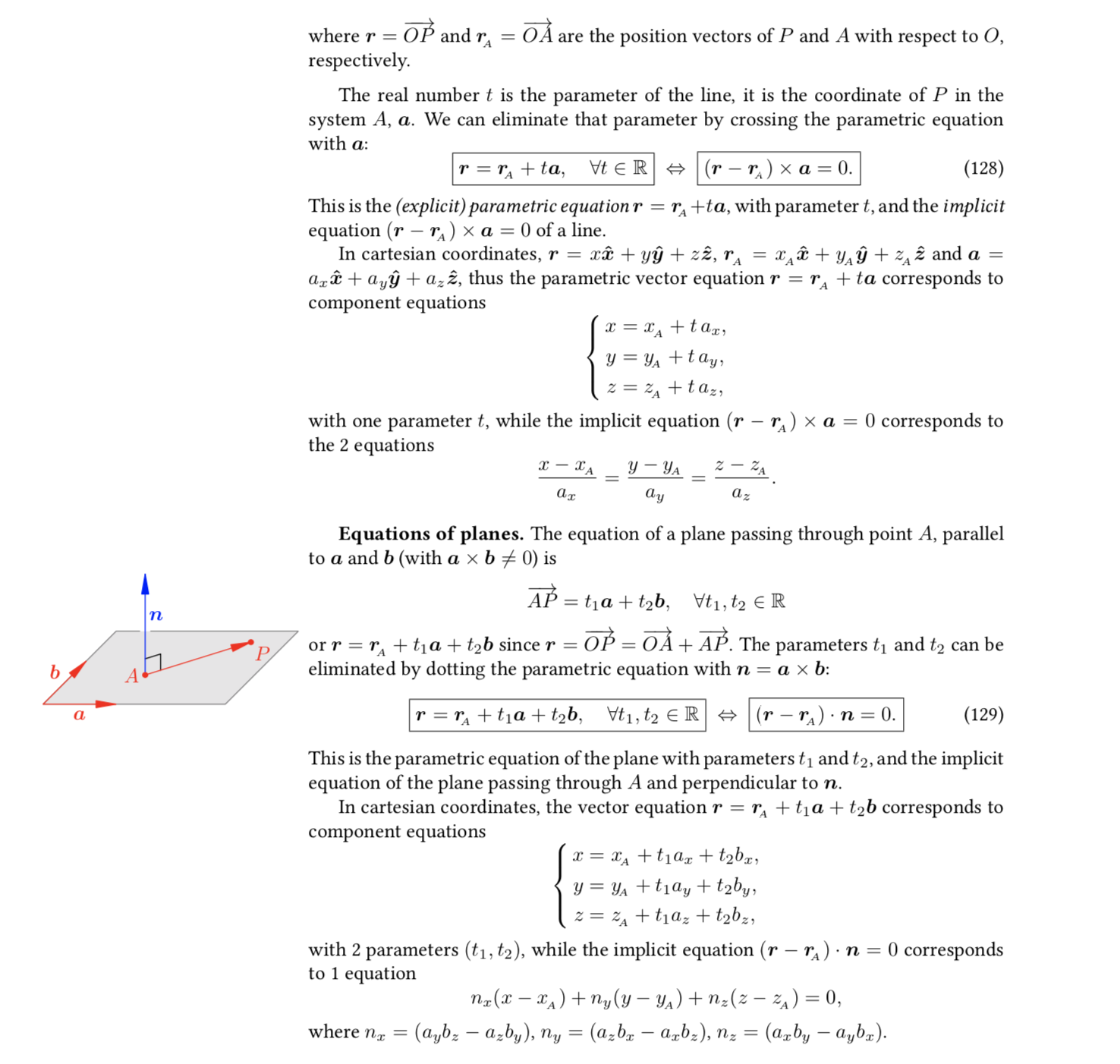
7. A particle was at point P1 at time t1 and is moving at the constant velocity '01. Another particle was at P2 at t2 and is moving at the constant velocity '02. How close did the particles get to each other and at What time? What conditions are needed for a collision? 8. Point 0 is obtained by rotating point B about the axis passing through point A, with direction a, by angle a (right hand rotation by a about (I). Find an explicit vector expression for C? in terms of 0?, Oj, a, and a. Make clean sketches. Express your vector result in cartesian form. Equations of lines. The vector equation of a line parallel to a passing through a point A is A_P)=ta,, VtE R (126) This vector equation expresses that the vector E is parallel to a, where t is a real parameter. In terms of an origin 0 we have 075 = Oj + [ and we can write the vector (parametric) equation of that same line as r = 'rA + t a, (127) where r = OP and r, = OA are the position vectors of P and A with respect to O, respectively. The real number t is the parameter of the line, it is the coordinate of P in the system A, a. We can eliminate that parameter by crossing the parametric equation with a: r = rAtta, WER (r - r ) x a = 0. (128) This is the (explicit) parametric equationr = rA +ta, with parameter t, and the implicit equation (r - TA ) x a = 0 of a line In cartesian coordinates, r = xx + yy + zz, TA = XAX + yAy + z, 2 and a = arx + ayy + azz, thus the parametric vector equation r = rA + ta corresponds to component equations y = yA + tay, z = ZA +taz, with one parameter t, while the implicit equation (r - " ) x a = 0 corresponds to the 2 equations X - TA y - yA - 2 - ZA ay a z Equations of planes. The equation of a plane passing through point A, parallel to a and b (with a x b # 0) is n AP = tia + tab, Vt1, t2 ER P orr = r, + tia + t2b sincer = OP = OA + AP. The parameters t] and t2 can be b eliminated by dotting the parametric equation with n = a x b: a r = "A + tia + tab, Vt1, t2 ER|4 (r-") . n=0. (129) This is the parametric equation of the plane with parameters to and t2, and the implicit equation of the plane passing through A and perpendicular to n. In cartesian coordinates, the vector equation r = TA + tia + t2b corresponds to component equations x = "A +tax + t2bx, y = ya +tidy + tzby, 2 = ZA + tiaz + t2bz, with 2 parameters (t1, t2), while the implicit equation (r - TA ) . n = 0 corresponds to 1 equation no(X - XA) + ny (y - yA ) + nz ( 2 - ZA ) = 0, where no = (aybz - azby), ny = (a=br - axbz), nz = (amby - dybz)