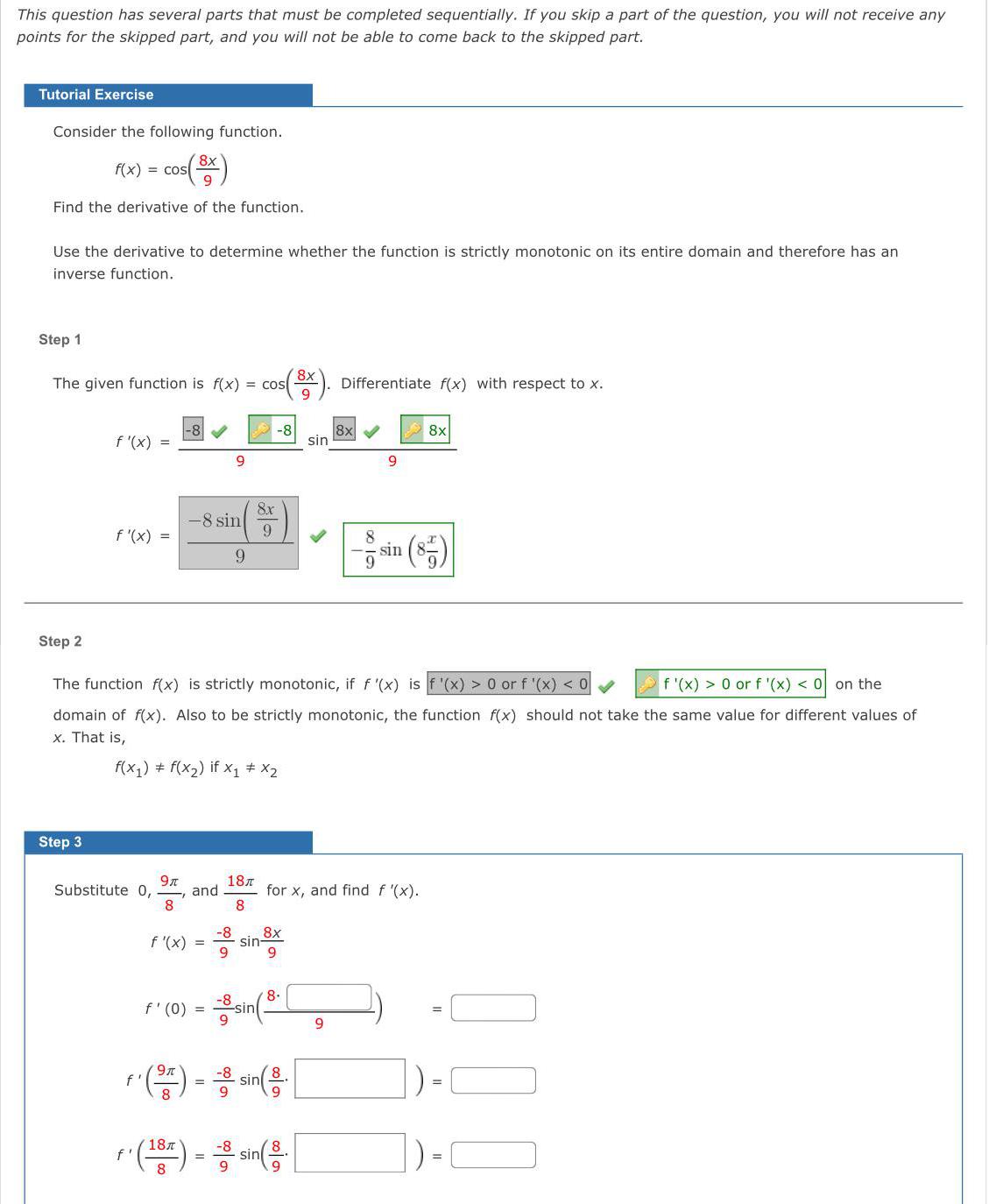
This question has several parts that must be completed sequentially. If you skip a part of the question, you will not receive any points for the skipped part, and you will not be able to come back to the skipped part. Tutorial Exercise Consider the following function. f(x) = cos 8x 9 Find the derivative of the function. Use the derivative to determine whether the function is strictly monotonic on its entire domain and therefore has an inverse function. Step 1 The given function is f(x) f'(x) = 9 = cos -8 (B). sin Differentiate f(x) with respect to x. 8x 8x 9 8x -8 sin f'(x) = 9 8 sin 9 Step 2 The function f(x) is strictly monotonic, if f'(x) is f'(x) > 0 or f'(x) < 0 f'(x)>0 or f'(x) < 0 on the domain of f(x). Also to be strictly monotonic, the function f(x) should not take the same value for different values of x. That is, f(x1) f(x2) if x1 + x2 Step 3 Substitute 0, 9 8 18 and for x, and find f'(x). 8 -8 8x f'(x) = sin- 9 9 f'(0) = 9 8. sin 9 9 f*(3x) = -* sin(3. == 9 f' (187) = *sin(9.