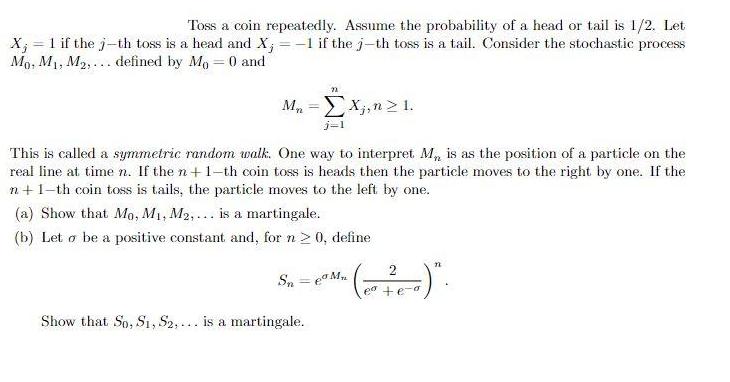
Toss a coin repeatedly. Assume the probability of a head or tail is 1/2. Let X;= = 1 if the j-th toss is a head and X; = -1 if the j-th toss is a tail. Consider the stochastic process Mo, M, M,... defined by Mo = 0 and 22 Mn - X;,n > 1. 72 j=1 This is called a symmetric random walk. One way to interpret M, is as the position of a particle on the real line at time n. If the n+1-th coin toss is heads then the particle moves to the right by one. If the n+1-th coin toss is tails, the particle moves to the left by one. (a) Show that Mo, M, M2,... is a martingale. (b) Let o be a positive constant and, for n 0, define Sn = eM Show that So, S1, S2,... is a martingale. 2 ea te-a 22