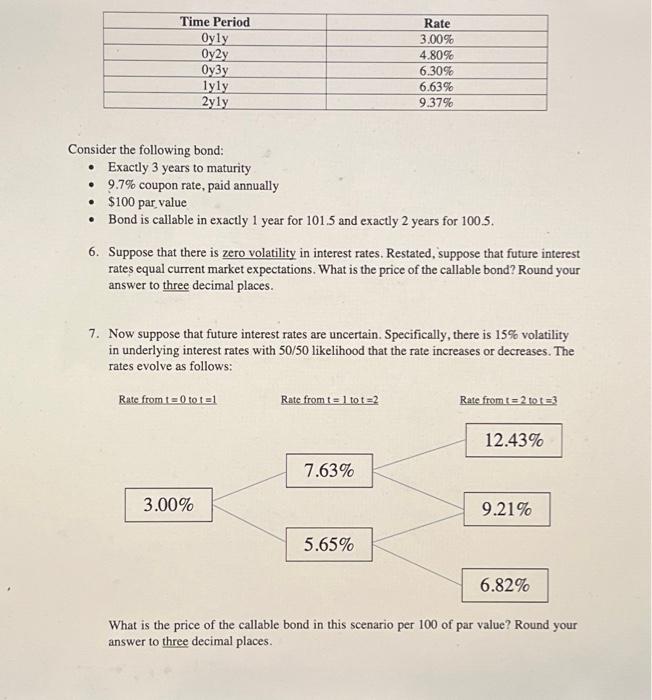
Use the following information for Questions 1-5: You observe the following set of spot rates: You also estimate that future interest rate volatility is 10%, which leads to the following binomial interest rate tree: 1. What is the value of a bond that matures in exactly 2 years, has par value of $100, an annual coupon of 4.4%, and is not callable? Round your answer to three decimal places. 2. Assume interest rates have 10% volatility as shown in the tree above. What is the value of a bond identical that of Problem \#1 but is callable in 1 year at a price of 101 ? Round your answer to three decimal places. 3. Relative to your answer in #2, what would happen to the price of the bond if it was callable at par and why? a. Price will decrease investors get less cash flow if the bond is called b. Price will decrease because the bond is less likely to get called c. Price will decrease because the bond is more likely to get called d. Price will decrease because investors get more cash flow if the bond is called c. Not enough information to answer 4. Relative to your answer in #2, what would happen to the callable bond's price if the future interest rate volatility was 5% rather than 10% and why? a. Price will decrease because the bond is less likely to get called b. Price will decrease because the bond is more likely to get called c. Price will increase because the bond is more likely to get called d. Price will increase because bond is less likely to get called e. Not enough information to answer 5. Relative to your answer in \#2, what would happen to the callable bond's price if future interest rates were known with certainty (e.g., future interest rates equal current expectations) and why? a. Price will decrease because the bond is less likely to get called b. Price will decrease because the bond is more likely to get called c. Price will increase because the bond is more likely to get called d. Price will increase because bond is less likely to get called e. Not enough information to answer Consider the following bond: - Exactly 3 years to maturity - 9.7% coupon rate, paid annually - \$100 par value - Bond is callable in exactly 1 year for 101.5 and exactly 2 years for 100.5 . 6. Suppose that there is zero volatility in interest rates. Restated, suppose that future interest rates equal current market expectations. What is the price of the callable bond? Round your answer to three decimal places. 7. Now suppose that future interest rates are uncertain. Specifically, there is 15% volatility in underlying interest rates with 50/50 likelihood that the rate increases or decreases. The rates evolve as follows: What is the price of the callable bond in this scenario per 100 of par value? Round your answer to three decimal places. 8. What is the investor's annualized rate of return on the callable bond if interest rates increase in both 1 and 2 years (e.g., the 1 -year rate at time 1 is 7.63%, the 1 -year rate at time 2 is 12.43%) ? Express your answer as a percentage and round your answer to three decimal places. 9. What is the investor's annualized rate of return on the callable bond if interest rates decrease in both 1 and 2 years (e.g., the 1 -year rate at time 1 is 5.65%, the 1 -year rate at time 2 is 6.82%) ? Express your answer as a percentage and round your answer to three decimal places. 10. Which types of firms would you expect to issue callable bonds and why? a. Riskier, younger firms because they have higher interest rates initially and thus more room for them to fall b. Riskier, younger firms because investors prefer these firms c. Older, safter firms because they have more room for their interest rates to increase d. Older, safer firms because their interest rates are lower