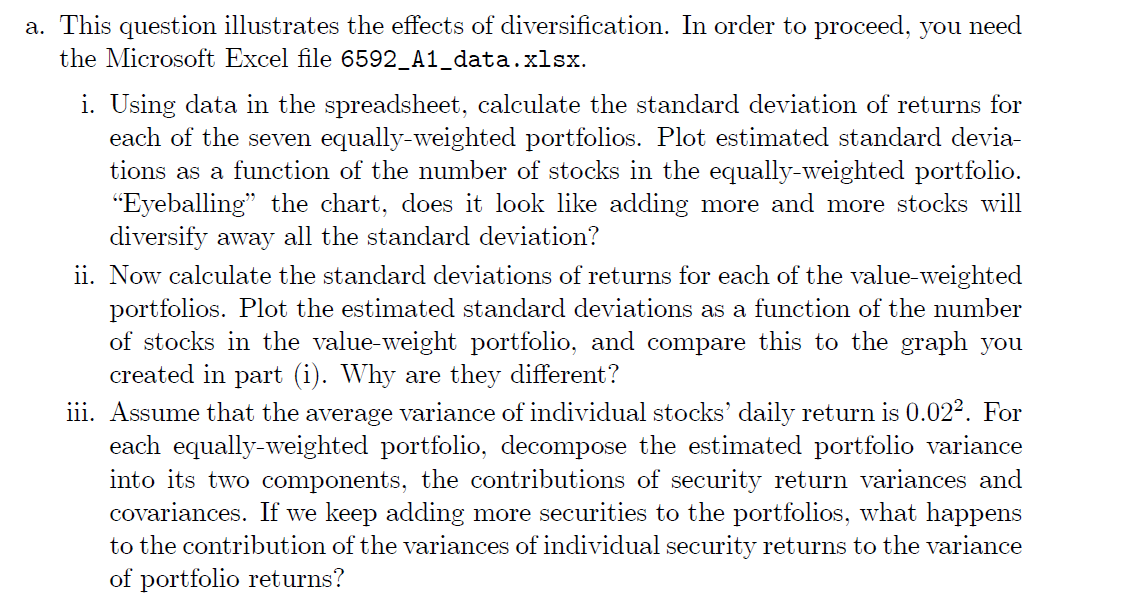
USE this link to get the DATA of for this questions: https://docs.google.com/spreadsheets/d/16bUfFyugeBm6kzHaWpL2j1c7sEaTCDEzQhaxgVLrEus/edit?usp=sharing

a. This question illustrates the effects of diversification. In order to proceed, you need the Microsoft Excel file 6592_A1_data.xlsx. i. Using data in the spreadsheet, calculate the standard deviation of returns for each of the seven equally-weighted portfolios. Plot estimated standard devia- tions as a function of the number of stocks in the equally-weighted portfolio. "Eyeballing" the chart, does it look like adding more and more stocks will diversify away all the standard deviation? ii. Now calculate the standard deviations of returns for each of the value-weighted portfolios. Plot the estimated standard deviations as a function of the number of stocks in the value-weight portfolio, and compare this to the graph you created in part (i). Why are they different? iii. Assume that the average variance of individual stocks' daily return is 0.022. For each equally-weighted portfolio, decompose the estimated portfolio variance into its two components, the contributions of security return variances and covariances. If we keep adding more securities to the portfolios, what happens to the contribution of the variances of individual security returns to the variance of portfolio returns? a. This question illustrates the effects of diversification. In order to proceed, you need the Microsoft Excel file 6592_A1_data.xlsx. i. Using data in the spreadsheet, calculate the standard deviation of returns for each of the seven equally-weighted portfolios. Plot estimated standard devia- tions as a function of the number of stocks in the equally-weighted portfolio. "Eyeballing" the chart, does it look like adding more and more stocks will diversify away all the standard deviation? ii. Now calculate the standard deviations of returns for each of the value-weighted portfolios. Plot the estimated standard deviations as a function of the number of stocks in the value-weight portfolio, and compare this to the graph you created in part (i). Why are they different? iii. Assume that the average variance of individual stocks' daily return is 0.022. For each equally-weighted portfolio, decompose the estimated portfolio variance into its two components, the contributions of security return variances and covariances. If we keep adding more securities to the portfolios, what happens to the contribution of the variances of individual security returns to the variance of portfolio returns