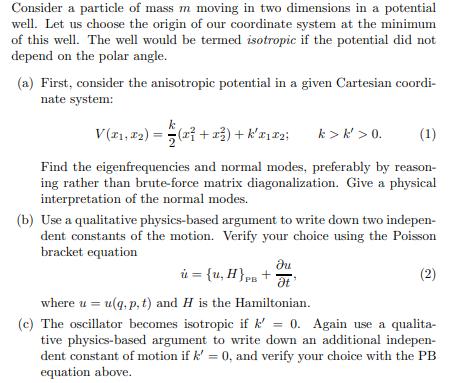
Consider a particle of mass m moving in two dimensions in a potential well. Let us choose the origin of our coordinate system at the minimum of this well. The well would be termed isotropic if the potential did not depend on the polar angle. (a) First, consider the anisotropic potential in a given Cartesian coordi- nate system: V(x,)=(x+x)+kxx; k> k' > 0. (1) Find the eigenfrequencies and normal modes, preferably by reason- ing rather than brute-force matrix diagonalization. Give a physical interpretation of the normal modes. (b) Use a qualitative physics-based argument to write down two indepen- dent constants of the motion. Verify your choice using the Poisson bracket equation = {u, H}PB + Ju t (2) where u = u(q, p, t) and H is the Hamiltonian. (c) The oscillator becomes isotropic if k' = 0. Again use a qualita- tive physics-based argument to write down an additional indepen- dent constant of motion if k' = 0, and verify your choice with the PB equation above.