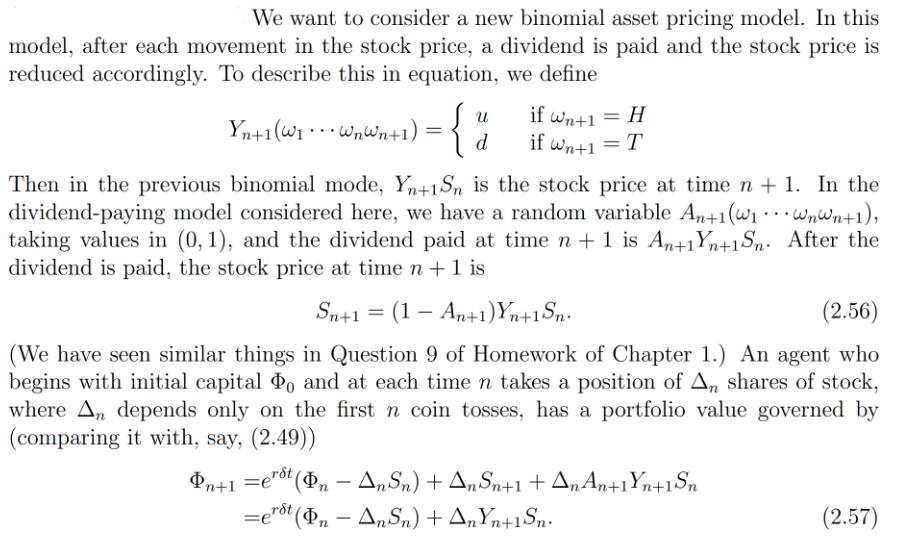
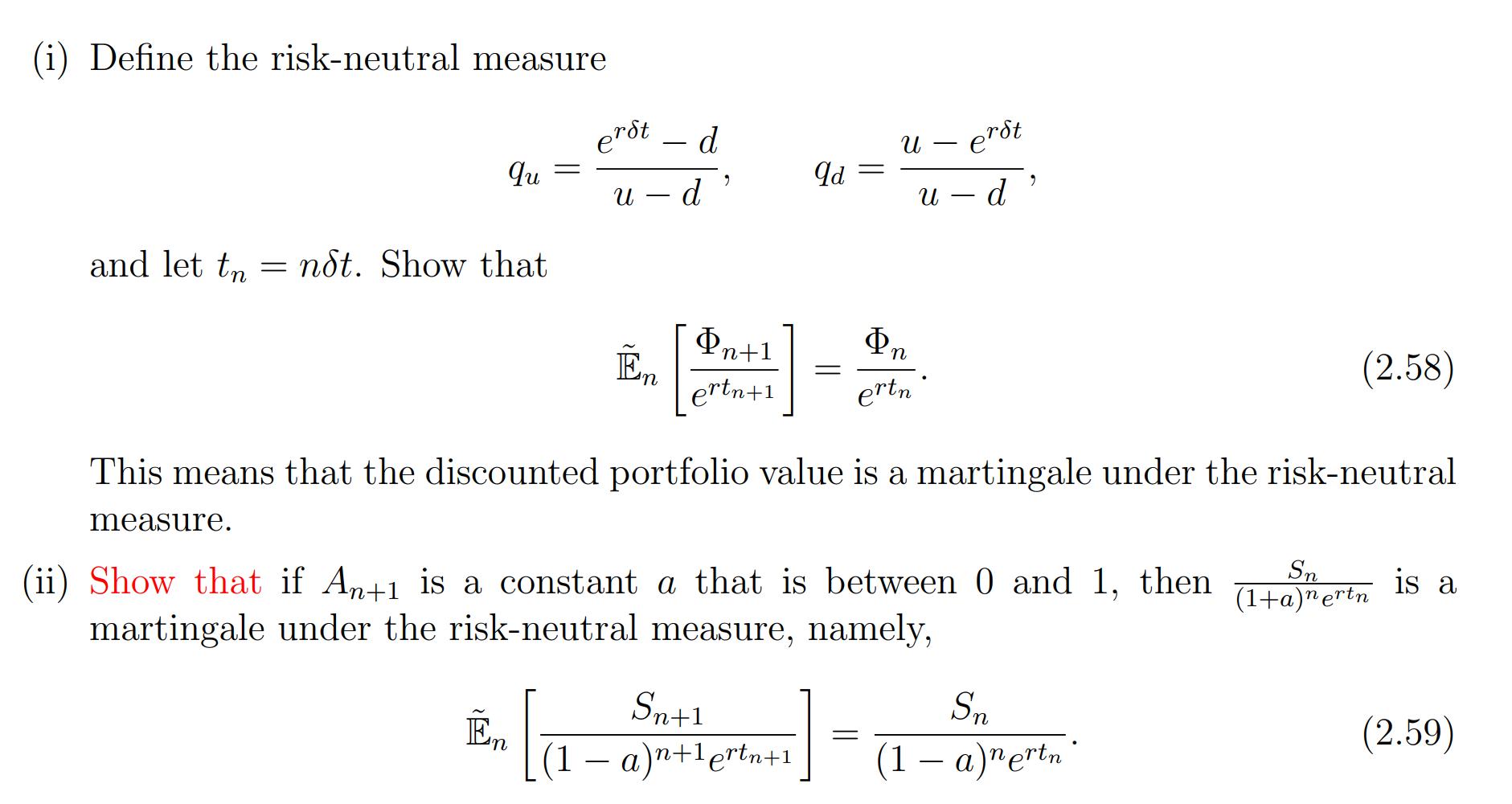
We want to consider a new binomial asset pricing model. In this model, after each movement in the stock price, a dividend is paid and the stock price is reduced accordingly. To describe this in equation, we define Yn+1 (W... WnWn+1): = {} d if wn+1 = H if Wn+1 = T Then in the previous binomial mode, Yn+1Sn is the stock price at time n + 1. In the dividend-paying model considered here, we have a random variable An+1(w Wnwn+1), taking values in (0, 1), and the dividend paid at time n + 1 is An+1Yn+1Sn. After the dividend is paid, the stock price at time n + 1 is Sn+1 = (1 - An+1) Yn+1 Sn. (2.56) (We have seen similar things in Question 9 of Homework of Chapter 1.) An agent who begins with initial capital Po and at each time n takes a position of An shares of stock, where An depends only on the first n coin tosses, has a portfolio value governed by (comparing it with, say, (2.49)) - n+1 =erst (n - AnSn) + AnSn+1+An An+1 Yn+1Sn =e erst (In AnSn) + AnYn+1Sn. ... (2.57) (i) Define the risk-neutral measure qu and let tn ndt. Show that = = ert En d U d n 9 Pn+1 ertn+1 qd = Sn+1 (1 a)n+1ertn+1 = U ertn = U Dn erst d This means that the discounted portfolio value is a martingale under the risk-neutral measure. (ii) Show that if An+1 is a constant a that is between 0 and 1, then martingale under the risk-neutral measure, namely, 9 Sn (2.58) a)nertn Sn (1+a)nertn is a (2.59)