A system is represented by the following ordinary differential equation where y(t)is the system output and x(t)is
Question:
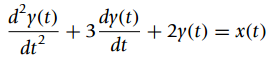
where y(t)is the system output and x(t)is the input.
(a) Find the transfer function H(s) = Y(s)/X(s)of the system. From its poles and zeros determine if the system is BIBO stable or not.
(b) If x(t) = u(t), and initial conditions are zero, determine the steady-state response yss(t). What if the initial conditions were not zero, would you get the same steady state? Explain.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





