Let S 1 2 and S 2 2 be the variances of independent random samples of sizes
Question:
Let S12 and S22 be the variances of independent random samples of sizes n1 and n2 selected from normally distributed populations with parameters (μ1, σ2) and (μ2, σ2), respectively. Thus, the populations have different means, but a common variance σ2. To estimate the common variance, we can combine information from both samples and use the pooled estimator
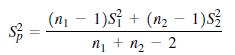
Use Theorems 6.11 and 6.12 to show that (n1 + n2 - 2)Sp2/σ2 has a chi-square distribution with v = (n1 + n2 - 2) degrees of freedom.
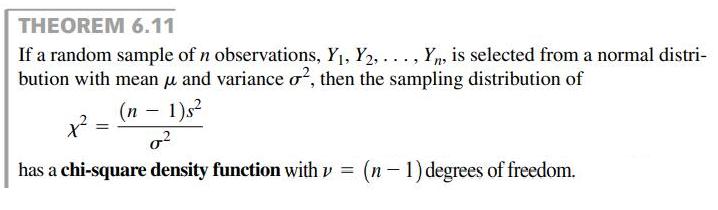
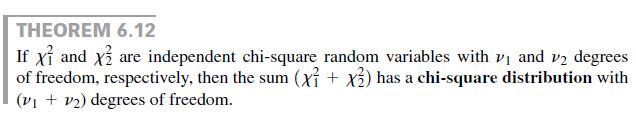
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Statistics For Engineering And The Sciences
ISBN: 9781498728850
6th Edition
Authors: William M. Mendenhall, Terry L. Sincich
Question Posted:





