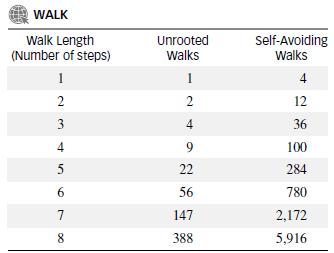
The American Scientist (July–Aug. 1998) published a study of the relationship between self-avoiding and unrooted walks. In a self-avoiding walk you never retrace or cross your own path, whereas an unrooted walk is a path in which the starting and ending points are impossible to distinguish. The possible number of walks of each type of various lengths are recorded in the accompanying table. Suppose you want to model the number of unrooted walks (y) as a function of walk length (x). Consider the quadratic model, E(y) = β_o + β₁x + β₂x². Is there sufficient evidence of an upward concave curvilinear relationship between y and x? Test at α = .10.

Transcribed Image Text:

WALK walk Length (Number of steps) Unrooted Walks Self-Avoiding Walks 1 4 2 12 3 4 36 4 9 100 5 22 284 6 56 780 7 147 2,172 8. 388 5,916