A pediatrician wants to - determine the relation that may exist between a child's height and head circumference. She randomly selects 11 children from her practice, measures their heights and head circumferences, and obtains the following data:

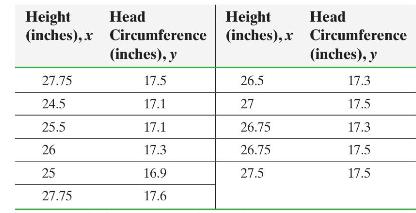
(a) Treating height as the explanatory variable, \(x\), determine the estimates of \(\beta_{0}\) and \(\beta_{1}\).

(b) Compute the standard error of the estimate, \(s_{e}\).

(c) Determine whether the residuals are normally distributed.

(d) Determine \(s_{b_{1}}\)

(e) If the residuals are normally distributed, test whether a linear relation exists between height and head circumference at the \(\alpha=0.01\) level of significance.

(f) If the residuals are normally distributed, construct a \(95 \%\) confidence interval about the slope of the true least-squares regression line.

(g) A child comes in for a physical, and the nurse determines his height to be 26.5 inches. However, the child is being rather uncooperative, so the nurse is unable to measure the head circumference of the child. What would be a good estimate of this child's head circumference? Why is this a good estimate?

Transcribed Image Text:

Height Head Height Head (inches), x Circumference (inches), x Circumference (inches), y (inches), y 27.75 17.5 26.5 17.3 24.5 17.1 27 17.5 25.5 17.1 26.75 17.3 26 17.3 26.75 17.5 25 16.9 27.5 17.5 27.75 17.6