An analyst is investigating the effect of certain policy events on common stock prices in a given

Question:

An analyst is investigating the effect of certain policy events on common stock prices in a given industry. In an attempt to isolate abnormal from normal returns of firms in the industry, the following returns-generating equation was estimated via least squares: \(R_{t}=\beta_{1}+\beta_{2} R_{m t}+\varepsilon_{t}\) where \(R_{t}\) is actual return by a firm on day \(t\) and \(R_{m t}\) is the return on a large portfolio of stocks designed to be representative of market return on day \(t\). Abnormal returns is defined to be \(\mathrm{AR}_{t}=\varepsilon_{t}\), and is estimated from an estimate of the returns-generating equation as

\(\widehat{\mathrm{AR}}_{t}=\mathrm{R}_{t}-\hat{\mathrm{R}}_{t}, \quad\) where \(\hat{R}_{t}=b_{1}+b_{2} R_{m t}\).

Thus, the estimated abnormal returns are effectively the estimated residuals from the least squares estimate of the returns generating equation. A summary of the estimation results from an analysis of 43 observations on a given firm is given below:

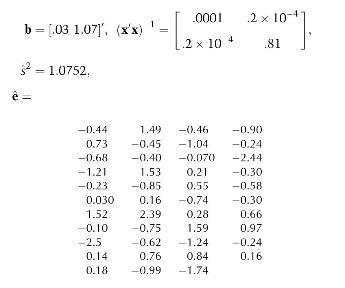
image text in transcribed

(a) Use a runs test to provide an indication of whether the residuals of the returns generating equation, and hence the abnormal returns, can be viewed as iid from some population distribution. Use a size .05 test. Comments on the appropriateness of the runs test in this application.

(b) Test to see whether the abnormal returns can be interpreted as a random sample from some normal population distribution of returns. Use a size .05 level of test.

(c) Assuming normality, test whether the expected daily return of the firm is proportional to market return. Use a size .05 test.

(d) Define and test a hypothesis that will assess whether the expected return of the firm is greater than market return for any \(R_{m t} \geq 0\). Use a size .05 test.

Fantastic news! We've Found the answer you've been seeking!