Exercise D.30 examines a relationship between relative age in a class and likelihood of ADHD diagnosis for boys in British Columbia. Girls are less likely overall to be diagnosed with ADHD but does the same relationship exist with relative age in school? Table D.13 shows the number of girls diagnosed with ADHD based on the quarter of the year in which they were born, as well as the proportion of girls born during that quarter. Answer the same questions as in Exercise D.30, using the data for girls instead of boys.

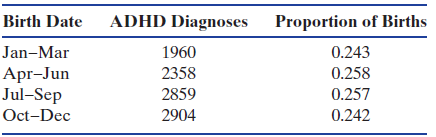
Table D.13

A new study indicates that the youngest children in a school grade are more likely to be diagnosed with attention-deficit/hyperactivity disorder (ADHD) than their older peers in the same grade. The study involved 937,943 children between 6 and 12 years old in British Columbia, Canada. The cutoff date for entering school in any year in British Columbia is December 31, so in any given class, those born late in the year are almost a year younger than those born early in the year. Is it possible that the younger students are being over-diagnosed with ADHD?

Exercise D.30

Table D.12 shows the number of boys diagnosed with ADHD based on the quarter of the year in which they were born, as well as the proportion of boys born during that quarter.

Table D.12

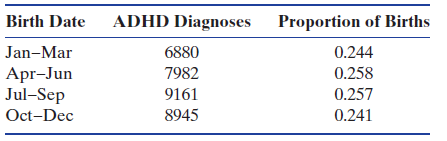
(a) What is the total number of boys diagnosed with ADHD in the sample?
(b) For the null hypothesis, use the overall proportion of births in a quarter to give the null proportion for that quarter. Compute the expected number of ADHD diagnoses for each quarter under this hypothesis.
(c) Compute the χ²-statistic.
(d) Give the degrees of freedom and find the p-value.
(e) State the conclusion of the test. For which group of children does ADHD appear to be diagnosed more frequently than we would expect? Less frequently? Write a sentence explaining what this means about ADHD and relative age in school.

ADHD Diagnoses Birth Date Proportion of Births 0.243 Jan-Mar Apr-Jun Jul-Sep 1960 2358 2859 2904 0.258 0.257 0.242 Oct-Dec Birth Date ADHD Diagnoses Proportion of Births Jan-Mar 6880 7982 9161 8945 0.244 Apr-Jun Jul-Sep Oct-Dec 0.258 0.257 0.241