Consider a particle of mass m moving in two dimensions, bound in a central potential V(r), so that its Hamiltonian is H = T(p) + V(r), where T(p) = (p²_x+ p²_y)/2m. Show that the quantity D = xp_x + yp_y is not a constant of the motion by computing D? = {D, H}.Compute D? for the special case that V(r) is a power law, V(r) = V₀r^a. Integrate your result over a time long compared to the period of this bound particle and show that 2? T ? = a? T(r) ? (where ? ?. ? denotes time averaging). This is the virial theorem of classical mechanics. Combine your result with energy conservation to express the total energy of the system in terms of ? T ?. What are the implications for the motion of the Moon around Earth eqs. (31.29)?

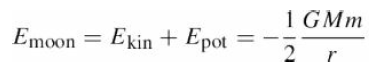
and (31.30)?

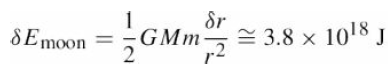
? you will need to use r?/?r = x?/?x + y?/?y and similar relations.

Transcribed Image Text:

1 GMm Emoon = Ekin + Epot %3D 2 r