An ideal gas characterised by the coefficient c found in relation (5.62) and the coefficient γ = (c + 1) /c undergoes a refrigeration cycle consisting of four reversible processes (Fig. 7.24):• 1−→ 2 : adiabatic compression• 2−→ 3 : isobaric compression• 3−→ 4 : isochoric cooling• 4−→ 1 : isobaric expansionAnalyse this cycle by using the following instructions: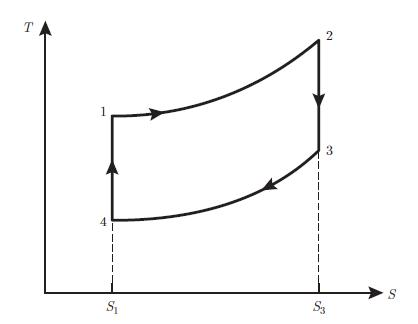

(T, S) diagram of a Rankine cycle operated on an ideal gasa) Determine the volume V2 in terms of the volumes V1 and V3 and the pressures p1 and p2.b) Find the entropy variation ΔS23 during the isobaric compression.c) Determine the heat exchanged Q23 during the isobaric compression.d) Assume now that instead of an ideal gas a fluid is used, which is entirely in a gaseous state at point 2 and completely in a liquid state at point 3. The isobaric compression 2 −→ 3 is then a phase transition occurring at temperature T and characterised by the molar latent heat of vaporisation g. Determine the entropy variation ΔS23 during the phase transition in terms of the number of moles N of fluid, the volume V2, the pressure p2 and the molar latent heat of vaporisation g, assuming that pV = NR T in the gas phase.

Transcribed Image Text:

T 1 4 S₁ 2 1 1 3 S3 S