Question: The flow field about a line source considers the symmetric radial flow of an incompressible, inviscid fluid outward from an infinitely long uniform source, coincident

The flow field about a line source considers the symmetric radial flow of an incompressible, inviscid fluid outward from an infinitely long uniform source, coincident with the z-axis of a cylindrical coordinate system. Fluid is being generated at a volumetric rate F per unit length of source.

(a) Show that the Laplace equation for the velocity potential for this system is

(b) From this equation find the velocity potential, velocity, and pressure as functions of position: where P_∞ is the value of the modified pressure far away from the source.

(c) Discuss the applicability of the results in (b) to the flow field about a well drilled into a large body of porous rock.

(d) Sketch the flow net of streamlines and equipotential lines.

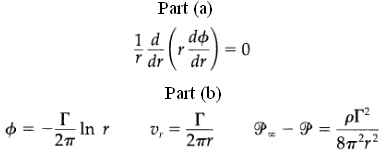
Part (a) dф) r dr dr Part (b) pГ? 8п?r2 Pz – P = In r 2т г Г v, = 2Tr

Part (a) d) r dr dr Part (b) p? 8?r2 Pz P = In r 2 v, = 2Tr

Step by Step Solution

★★★★★

3.53 Rating (180 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

The flow field around a line source a For this pur... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Document Format (1 attachment)

6-E-C-E-T-P (86).docx

120 KBs Word File

Students Have Also Explored These Related Chemical Engineering Questions!

An infinitely long line charge having a uniform charge per unit length A lies a distance d from point O as shown in Figure P24.19. Determine the total electric flux through the surface of a sphere of...

An incompressible fluid with density p is in a horizontal test rube of inner cross-sectional area. The test tube spins in a horizontal circle in an ultracentrifuge at an angular speed w....

An infinitely long line of charge with uniform density l = 9 (nC/m) lies in the xy plane parallel to the y-axis at x _ 2 m. Find the potential VAB at point A (3m, 0, 4m) in Cartesian coordinates with...

For this assignment, you will write a program to find a maximum flow in a flow network. User Requirements The user is a start-up software company that specializes in software for combinatorial...

Republic of Iraq Ministry of Higher Education and Scientific Research University of Technology Chemical Engineering Department MODELING AND SIMULATION OF FCC RISERS A RESEARCH SUBMITTED TO THE...

Exercise 1. Going back to Section 16.2, read the subsection "Gradient Fields" (p. 977 - 978). According to this reading, what physical quantity does the potential function f represent in many...

Converging duct flow is modeled by the steady, two-dimensional velocity field of Prob. 417. Since the flow is symmetric about the x-axis, line segment AB along the x-axis remains on the axis, but...

Please help me .. am stuck... please address all questions clearly:- The power rating of a motor can be modified to account for different ambient temperature, according to the following table:...

Pool 4 . 3 Write down the complex potential for a source of strength m located at z = i h and a source of strength m located at z = - i . Show that the real axis is a streamline in the resulting flow...

Problem A 2 D source of strength located at the origin ( 0 , 0 ) is put into a parallel flow with velocity u 0 in positive x - axis direction. The velocity potential of the flow is given by = u 0 x +...

International Beverages acquires Ecoplastics Recycling Company for $100,000,000 in cash. Ecoplastics balance sheet reports the following assets and liabilities, at fair value: Cash & receivables...

The oldest national daily newspaper in the United States is A. the New York Times. B. the Wall Street Journal. C. the Christian Science Monitor. D. USA Today

The shipping department of an appliances manufacturer in Chicago works three shifts, 24 hours a day. The department has two loading stations to load products (e.g., refrigerators, washers, dryers) to...

Which of the following does not declare a 2-by-2 array and set all four of its elements to 0? Select one: O a. int b[ 2 ][ 2 ]: for ( int i = 0; i <: 2; i++) for ( int j = 0: j <: 2: j++ ) b[ i...

40 What will be the output after the following C++ statements have been executed? // Good Luck out of I/ welcome mainol int rows=2: forint i= 1; i

- 13 A program can have the same name for local and global variables but the value of a global variable inside a function will take preference. ed out of Select one: O a. False question O b. True

estion 34 ot yet swered What will be the output after the following C++ statements have been executed? #include using nmespace std:main0{int rows=2for(int i=1; i

Joseph L. Smith (age 45, Social Security number 145-26-9210) and Rita M. Smith (age 43, Social Security number 142-46-5108) are husband and wife. They live at 1650 Belmont Avenue, Chicago, IL 60615....

Calculate the height of a mercury column required to produce a pressure (a) Of 0.984 atm; (b) Of 928 Torr; (c) Equal to that of a column of water 142 ft high.

The IS curve shows the equilibrium combinations of the real interest rate and real GDP. a. Demonstrate using graphs how the IS curve represents equilibrium in the goods market. b. Now suppose that...

For each of the following changes, identify (1) whether there is a shift in the IS curve or a movement along the curve, and (2) if the curve shifts, state the direction in which it shifts. a. The...

Consider the following statement: The IS curve slopes downward because a fall in short-term nominal interest rates increases the money supply and decreases investment spending. Do you agree with this...

The Great Depression began in the summer of 1929, but the stock market crash of October 1929 may have deepened the initial recession. The stock market crash increased the pessimism of both households...

During the 1960s, a major restructuring of the tax code decreased taxes for most people. Also during these years, the war in Vietnam required increased government purchases. a. Which components of...

Question 1 Suppose a voluntary retirement annuity scheme is launched for 65-year-old people. If a lump- sum premium of $1,000,000 is paid, the annuitant will receive $5,800 at the end of every month....