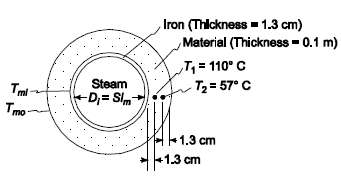
The thermal conductivity of a material may be determined in the following manner. Saturated steam 2.41 × 105 N/m2 is condensed at the rate of 0.68 kg/h inside a hollow iron sphere that is 1.3 cm thick and has an internal diameter of 51 cm. The sphere is coated with the material whose thermal conductivity is to be evaluated. The thickness of the material to be tested is 10 cm and there are two thermocouples embedded in it, one 1.3 cm from the surface of the iron sphere and one 1.3 cm from the exterior surface of the system. If the inner thermocouple indicates a temperature of 110?C and the outer themocouple a temperature of 57?C, calculate (a) the thermal conductivity of the material surrounding the metal sphere, (b) the temperatures at the interior and exterior surfaces of the test material, and (c) the overall heat transfer coefficient based on the interior surface of the iron sphere, assuming the thermal resistances at the surfaces, as well as the interface between the two spherical shells, are negligible.GIVENHollow iron sphere with saturated steam inside and coated with material outsideSteam pressure = 2.41 × 105 N/m2Steam condensation rate (ms ) = 0.68 kg/hInside diameter (Di) = 51 cm = 0.51 mThickness of the iron sphere (Ls) = 1.3 cm = 0.013 mThickness of material layer (Lm) = 10 cm = 0.1 mTwo thermocouples are located 1.3 cm from the inner and outer surface of the material layerInner thermocouple temperature (T1) = 110?COuter thermocouple temperature (T2) = 57?CASSUMPTIONSThermal resistance at the surface is negligibleThermal resistance at the interface is negligibleThe system has reached steady-stateThe thermal conductivities are constantOne dimensional conductionradially

Transcribed Image Text:

Iron (Thickness 1.3 cm) Material (Thickness - 0.1 m) T = 110° C T2 = 57° C Steam D,= Slm Tm - Tmo 1.3 cm +1.3 cm