We know that the charge on a conductor goes to the surface, but just how it distributes itself there is not easy to determine. One famous example in which the surface charge density can be calculated explicitly is the ellipsoid: In this case11 where Q is the total charge. By choosing appropriate values for a, b, and c, obtains (from Eq. 2.57):

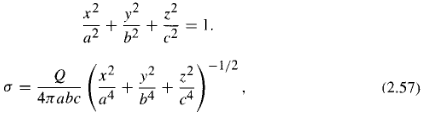
(a) The net (both sides) surface charge density o(r) on a circular disk of radius R;

(b) The net surface charge density o(x) on an infinite conducting "ribbon" in the x y plane, which straddles the y axis from x = -a to x: a (let A be the total charge per unit length of ribbon);

(c) The net charge per unit length k(x) on a conducting "needle", running from x = -a to x = a. In each case, sketch the graph of your result.

1. -1/2 4b q4 (2.57) 4