Width of diffraction maximum we suppose that in a linear crystal there are identical point scattering centers
Question:
Width of diffraction maximum we suppose that in a linear crystal there are identical point scattering centers at every lattice point pm = ma, where m is an integer. By analogy with (20), the total scattered radiation amplitude will be proportional of F = Σ exp [– ima ∙ Δk]. The sum over M lattice points is F = 1 – exp [–iM(a ∙ Δk]/ 1 – exp[–i(a ∙ Δk)], by the use of the series
(a) The scattered intensity is proportional to |F|2. Show that |F|2 ≡ F∙F = sin2 ½ M (a ∙ ΔK)/sin2 ½ (a ∙ Δk)
(b) We know that a diffraction maximum appears when a ∙ Δk = 2πh, where h is an integer, we change Δk slightly and define ε in a ∙ Δk = 2πh + ε such that ε gives the position of the first zero in sin ½ M(a ∙ Δk). Show that ε = 2π/M, so that the width of the diffraction maximum is proportional to 1/M and can be extremely narrow for macroscopic values of M. The same result holds true for a three-dimensional crystal.
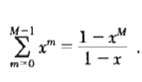
Step by Step Answer:






