Davison Electronics manufactures two models of LCD televisions, identified as model A and model B. Each model

Question:

Davison Electronics manufactures two models of LCD televisions, identified as model A and model B. Each model has its lowest possible production cost when produced on Davison€™s new production line. However, the new production line does not have the capacity to handle the total production of both models. As a result, at least some of the production must be routed to a higher-cost, old production line. The following table shows the minimum production requirements for next month, the production line capacities in units per month, and the production cost per unit for each production line:
Production Cost per Unit New Line Old Line Minimum Production Requirements Model $30 $25 $50 50,000 70,000 $40 60,000 B

Production Cost per Unit New Line Old Line Minimum Production Requirements Model $30 $25 $50 50,000 70,000 $40 60,000 B

Let

AN = Units of model A produced on the new production line

AO = Units of model A produced on the old production line

BN = Units of model B produced on the new production line

BO = Units of model B produced on the old production line

Davison€™s objective is to determine the minimum cost production plan. The computer solution is shown in Figure 3.21.

a. Formulate the linear programming model for this problem using the following four constraints:

Constraint 1: Minimum production for model A

Constraint 2: Minimum production for model B

Constraint 3: Capacity of the new production line

Constraint 4: Capacity of the old production line

b. Using computer solution in Figure 3.21, what is the optimal solution, and what is the total production cost associated with this solution?

c. Which constraints are binding? Explain.

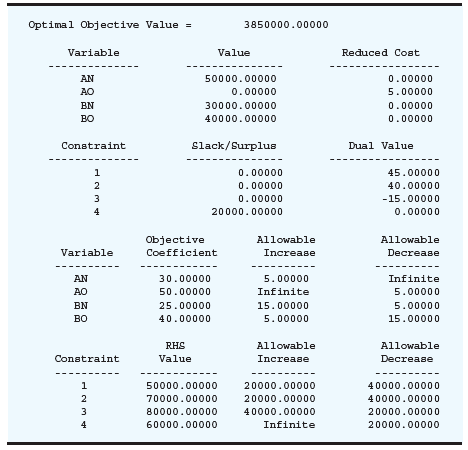
Optimal Objective Value = 3850000.00000 Variable Value Reduced Cost 50000.00000 0.00000 AN 0.00000 AO 5.00000 BN 30000.0

d. The production manager noted that the only constraint with a positive dual value is the constraint on the capacity of the new production line. The manager€™s interpretation of the dual value was that a one-unit increase in the right-hand side of this constraint would actually increase the total production cost by $15 per unit. Do you agree with this interpretation? Would an increase in capacity for the new production line be desirable? Explain.
e. Would you recommend increasing the capacity of the old production line? Explain.
f. The production cost for model A on the old production line is $50 per unit. How much would this cost have to change to make it worthwhile to produce model A on the old production line? Explain.
g. Suppose that the minimum production requirement for model B is reduced from 70,000 units to 60,000 units. What effect would this change have on the total production cost? Explain.