The Human Resources Department of a large company wishes to compare two methods of training industrial workers to perform a skilled task. Twenty workers are selected: 10 of them are randomly assigned to be trained using method \(\mathrm{A}\), and the other 10 are assigned to be trained using method B. After the training is complete, all the workers are tested on the speed of performance at the task. The times taken to complete the task are:

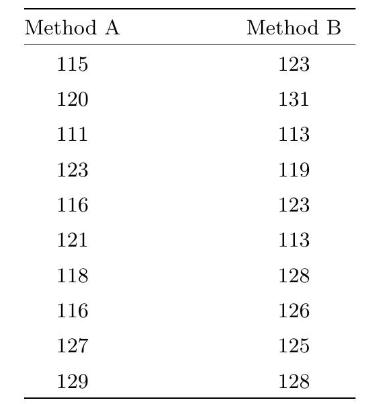
(a) We will assume that the observations come from \(\operatorname{normal}\left(A^{2}\right)\) and normal( \(\left.B_{B}^{2}\right)\), where \({ }^{2}=6^{2}\). Use independent normal \(\left(m s^{2}\right)\) prior distributions for \(A_{A}\) and \({ }_{B}\), respectively, where \(m=100\) and \(s^{2}=20^{2}\). Find the posterior distributions of \(A\) and \({ }_{B}\), respectively.
(b) Find the posterior distribution of \({ }_{A}{ }_{B}\).
(c) Find a \(95 \%\) Bayesian credible interval for \({ }_{A}{ }_{B}\).
(d) Perform a Bayesian test of the hypothesis
\[
H_{0}:{ }_{A} \quad B=0 \text { versus } H_{1}:{ }_{A} \quad{ }_{B}=0
\]
at the \(5 \%\) level of signi cance. What conclusion can we draw?

Transcribed Image Text:

Method A Method B 115 123 120 131 111 113 123 119 116 123 121 113 118 128 116 126 127 125 129 128