The spectral slope is an important characteristic of voices and musical instruments. The spectral slope is the slope \(b\) of the regression line between \(\ln x\) and \(y\), where \(x\) is the frequency (measured in hertz), and \(y\) is the amplitude (measured in decibels). You have made such measurements from a recording of a singer who sings the vowel \(\mathrm{E}\). The measurements are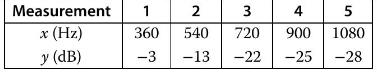

\(\sigma\) is unknown. Let \(z=\ln x\). We are going to look at the regression between \(z\) and \(y\).

(a) Find the regression line \(y=\alpha_{*}+\beta(z-\bar{z})\).

(b) Find the posterior probability distribution of the spectral slope \(b\).

(c) A mathematics savvy vocal afficionado claims that this particular singer has a greater spectral slope than -25 when he sings E. Determine this hypothesis with significance \(\alpha=0.1\).

Transcribed Image Text:

Measurement - 2 3 4 5 x (Hz) 360 540 720 900 1080 y (dB) -3 -13 -22 -25 -28