The equations r = a + b cos and r = a + b sin

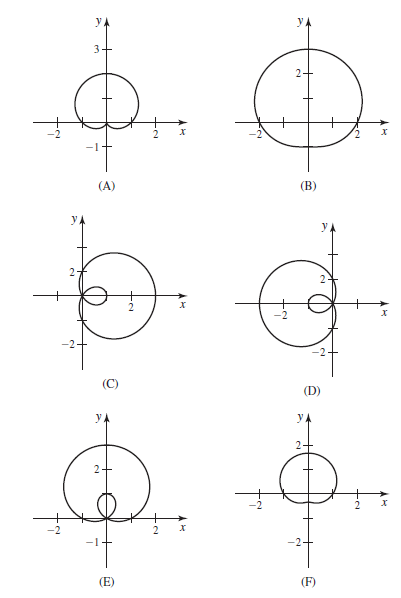
The equations r = a + b cos θ and r = a + b sin θ describe curves known as limaçons (from Latin for snail). We have already encountered cardioids, which occur when |a| = |b|. The limaçon has an inner loop if |a| < |b|. The limaçon has a dent or dimple if |b| < |a| < 2|b|. And the limaçon is oval-shaped if |a| > 2|b|. Match equations a–f with the limaçons in the figures A–F.

a. r = -1 + sin θ 

b. r = -1 + 2 cos θ

c. r = 2 + sin θ 

d. r = 1 - 2 cos θ

e. r = 1 + 2 sin θ 

f. r = 1 + 2/3 sin θ

Transcribed Image Text:

УА 2- х х (A) (B) У х х (C) (D) y. х х -1 (E) (F)