A differential condensation [see Eq. ( (9-15)) ] is done for a binary mixture of ethanol and

Question:

A differential condensation [see Eq. ( \(9-15)\) ] is done for a binary mixture of ethanol and water. The feed is \(0.50 \mathrm{kmol}\) of vapor that is \(10.0 \mathrm{~mol} \%\) ethanol. The differential condensation is continued until the vapor remaining is \(\mathrm{y}_{\mathrm{D}, \text { final }}=50.0 \mathrm{~mol} \%\) ethanol. Find the values of \(\mathrm{D}_{\text {fin }}\) and the condensate \(C_{\text {total }}\) and the average mole fraction of ethanol in the condensate, \(\mathrm{x}_{\mathrm{C}, \text { avg }}\). Note that this operation can only be approximated in practice (Treybal, 1980).

Equation 9-15

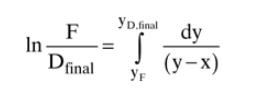
image text in transcribed