A bond has a quoted price of $1,080.42. It has a face value of $1,000, a semiannual coupon of $30, and a maturity of five years. What is its current yield? What is its yield to maturity? Which is bigger? Why?

Notice that this bond makes semiannual payments of $30, so the annual payment is $60.

The current yield is $60/$1,080.42 = .0555, or 5.55 percent. To calculate the yield to maturity, refer back to Example 8.1. Now, in this case, the bond pays $30 every six months and it has 10 six-month periods until maturity. So, we need to find R as follows:

Example 8.1

In practice, bonds issued in the United States usually make coupon payments twice a year. So, if an ordinary bond has a coupon rate of 14 percent, the owner will receive a total of $140 per year, but this $140 will come in two payments of $70 each.

Suppose the yield to maturity on our bond is quoted at 16 percent. Bond yields are quoted as annual percentage rates (APRs); the quoted rate is equal to the actual rate per period multiplied by the number of periods. With a 16 percent quoted yield and semiannual payments, the true yield is 8 percent per six months. If our bond matures in seven years, what is the bond’s price? What is the effective annual yield on this bond?

Based on our discussion, we know the bond will sell at a discount because it has a coupon rate of 7 percent every six months when the market requires 8 percent every six months. So, if our answer exceeds $1,000, we know that we have made a mistake.

To get the exact price, we first calculate the present value of the bond’s face value of $1,000 paid in seven years. This seven-year period has 14 periods of six months each. At 8 percent per period, the value is:

The coupons can be viewed as a 14-period annuity of $70 per period. At an 8 percent discount rate, the present value of such an annuity is: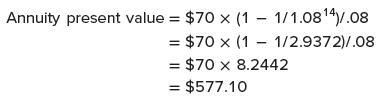

The total present value is the bond’s price:

Bond value = $340.46 + 577.10 = $917.56 To calculate the effective yield on this bond, note that 8 percent every six months is equivalent to:

Effective annual rate = ( 1 + .08 )² − 1 = .1664, or 16.64%

The effective yield, therefore, is 16.64 percent.

After some trial and error, we find that R is equal to 2.1 percent. But the tricky part is that this 2.1 percent is the yield per six months. We have to double it to get the yield to maturity, so the yield to maturity is 4.2 percent, which is less than the current yield. Why is the yield to maturity less than the current yield? The reason is that the current yield ignores the built-in loss of the premium between now and maturity.

Transcribed Image Text:

$1,080.42 $30 x [11/(1+ R) ¹01/R + $1,000/(1 + R) ¹0 10 =