17.11 Suppose that X and Y are distributed bivariate normal with density given in Equation (17.38).

a. Show that the density of Y given X = x can be written as

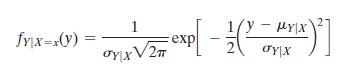
where sYX = 2s2 Y(1 - r2 XY) and mYX = mY - (sXY>s2 X)(x - mX).
[Hint: Use the definition of the conditional probability density fY 0X= x(y) = 3gX, Y(x, y)4 > 3fX(x)4, where gX,Y is the joint density of X and Y, and ƒX is the marginal density of X.]

b. Use the result in

(a) to show that Y0X = x N(mY 0X, s2 Y 0 X).

c. Use the result in

(b) to show that E(Y0X = x) = a + bx for suitably chosen constants a and b.

Transcribed Image Text:

1 fy|x=x(y) exp xV2 ( - - MYX