Hypergeometric Distribution If we sample from a small finite population without replacement, the binomial distribution should not be used because the events are not independent.

If sampling is done without replacement and the outcomes belong to one of two types, we can use the hypergeometric distribution. If a population has A objects of one type (such as lottery numbers matching the ones you selected), while the remaining B objects are of the other type (such as lottery numbers different from the ones you selected), and if n objects are sampled without replacement (such as six drawn lottery numbers), then the probability of getting x objects of type A and n - x objects of type B is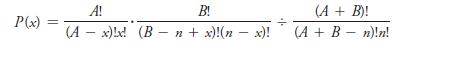

In Pennsylvania’s Match 6 Lotto game, a bettor selects six numbers from 1 to 49 (without repetition), and a winning six-number combination is later randomly selected. Find the probabilities of the following events and express them in decimal form.

a. You purchase one ticket with a six-number combination and you get exactly four winning numbers. (Hint: Use A = 6, B = 43, n = 6, and x = 4.)

b. You purchase one ticket with a six-number combination and you get all six of the winning numbers.

c. You purchase one ticket with a six-number combination and you get none of the winning numbers.

Transcribed Image Text:

A! B! P(x) = (A x)!! (B n + x)!(n - x)! (A + B)! (A + B - n)!n!