Consider steady, incompressible, two-dimensional shear flow for which the velocity field is where a and b are
Question:
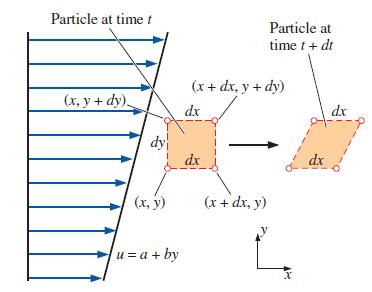
Consider steady, incompressible, two-dimensional shear flow for which the velocity field is
![]()
where a and b are constants. Sketched in Fig. P4–64 is a small rectangular fluid particle of dimensions dx and dy at time t. The fluid particle moves and deforms with the flow such that at a later time (t + dt), the particle is no longer rectangular, as also shown in the figure. The initial location of each corner of the fluid particle is labeled in Fig. P4–64. The lower-left corner is at (x, y) at time t, where the x-component of velocity is u = a + by. At the later time, this corner moves to (x + u dt, y), or (x + (a + by) dt, y)
(a) In similar fashion, calculate the location of each of the other three corners of the fluid particle at time t + dt.
(b) From the fundamental definition of linear strain rate (the rate of increase in length per unit length), calculate linear strain rates εxx and εyy.
(c) Compare your results with those obtained from the equations for εxx and εyy in Cartesian coordinates, i.e.,
![]()
Step by Step Answer:

Fluid Mechanics Fundamentals And Applications
ISBN: 9781259696534
4th Edition
Authors: Yunus Cengel, John Cimbala





