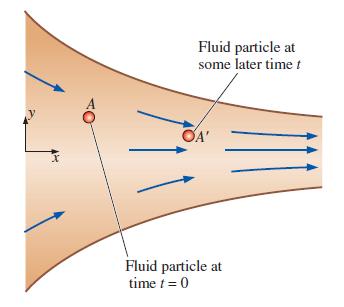
Converging duct flow is modeled by the steady, two dimensional velocity field of Prob. 4–17. A fluid particle (A) is located at x = x_A and y = y_A at time t = 0 (Fig. P4–54). At some later time t, the fluid particle has moved downstream with the flow to some new location x = x_A´, y = y_A´, as shown in the figure. Generate an analytical expression for the y-location of the fluid particle at arbitrary time t in terms of its initial y-location y_A and constant b. In other words, develop an expression for y_A´. 

Data from Problem 4-17.

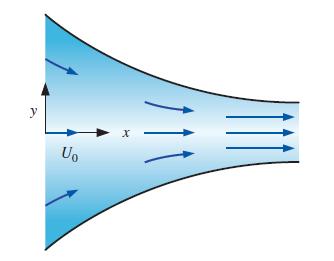
Consider steady, incompressible, two-dimensional flow through a converging duct (Fig. P4–17). A simple approximate velocity field for this flow is

where U₀ is the horizontal speed at x = 0. Note that this equation ignores viscous effects along the walls but is a reasonable approximation throughout the majority of the flow field. Calculate the material acceleration for fluid particles passing through this duct. Give your answer in two ways:

(1) As acceleration components a_x and a_y 

(2) As acceleration vector a(vector)

X Fluid particle at some later time t OA' Fluid particle at time t = 0