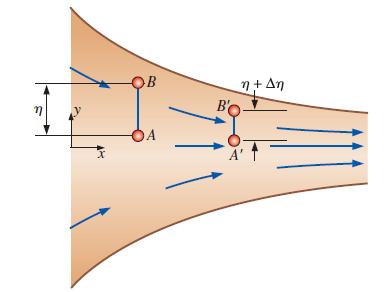
Using the results of Prob. 4–55 and the fundamental definition of linear strain rate (the rate of increase in length per unit length), develop an expression for the linear strain rate in the y-direction (ε_yy) of fluid particles moving down the channel. Compare your result to the general expression for ε_yy in terms of the velocity field, i.e., ε_yy = ∂ν/∂y.

Data from Problem 4-55

Converging duct flow is modeled by the steady, two dimensional velocity field. As vertical line segment AB moves downstream it shrinks from length η to length η + Δη as sketched in Fig. P4–55. Generate an analytical expression for the change in length of the line segment, Δη. Note that the change in length, Δη, is negative.

Transcribed Image Text:

n OA 3,+ An B'O