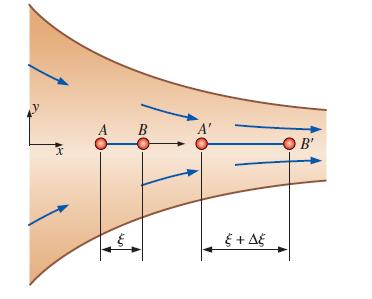
Converging duct flow is modeled by the steady, two-dimensional velocity field of Prob. 4–17. Since the flow is symmetric about the x-axis, line segment AB along the x-axis remains on the axis, but stretches from length ξ to length + 1 Δξ as it flows along the channel centerline (Fig. P4–52). Generate an analytical expression for the change in length of the line segment, Δξ.

Data from Problem 4-17.

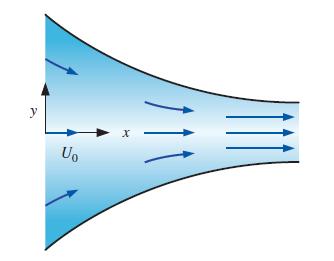
Consider steady, incompressible, two-dimensional flow through a converging duct (Fig. P4–17). A simple approximate velocity field for this flow is

where U₀ is the horizontal speed at x = 0. Note that this equation ignores viscous effects along the walls but is a reasonable approximation throughout the majority of the flow field. Calculate the material acceleration for fluid particles passing through this duct. Give your answer in two ways:

(1) As acceleration components a_x and a_y 

(2) As acceleration vector a(vector)

Transcribed Image Text:

A Un B A' { + Δέ Ο Β'