(a) Assume that f(t), in problem 4.9, is given by exp (t). Show that, in this case,...
Question:
(a) Assume that f(t), in problem 4.9, is given by exp (–αt). Show that, in this case, ξ(t) satisfies the Bessel equation of zero order,
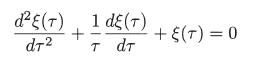
where τ = (Ωc/2α) exp (–αt). Determine the two solutions of this equation which satisfy the initial conditions stated in problem 4.9 and interpret them physically.
(b) Considering now that f(t) = ( 1 – αt), solve the equation for ξ(t) in problem 4.9 in a power series in α, and determine the particle trajectory to order α. Show that the ratio ( vx2 + vy2)/B(t) has no terms of order a, thus verifying the adiabatic in variance of the magnetic moment. Compare these results with those of problem 4.8.
Data from Problem 4.8.
Consider the motion of an electron in a spatially uniform magnetic field B = Bzẑ, such that Bz has a slow time variation given by
![]()
where B0 and α are positive constants, and |αt| ≪ 1. Assume the following
initial conditions: r(0) = (rc, 0, 0) and v(0) = (0, V⊥0, 0), where rc is the Larmor radius, V⊥0 = Ωcrc and Ωc = |q| B0/m.
Data from Problem 4.9.
Consider the motion of a charged particle in a spatially uniform magnetic field that varies slowly in time as compared to the particle cyclotron period.
Show that the equation of motion can be written in vector form as
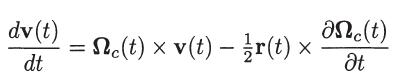
where Ωc(t) = –qB(t)/m.
Considering that B(t) = ẑB0f(t), where B0 is constant, obtain the following equations for the motion of the particle in the plane normal to B:
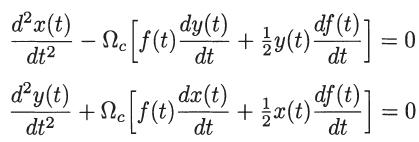
where Ωc = |q| B/m.
Define a complex variable u(t) = x(t) + iy(t) and a function ξ(t) by
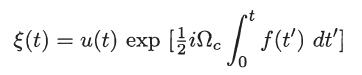
and show that the equation satisfied by ξ(t) is
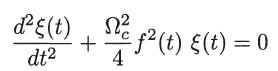
If ξ1(t) and ξ2(t) are two linearly independent solutions of this equation, subject to the initial conditions
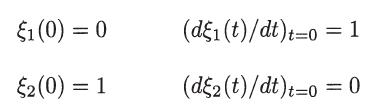
show that the solution for u(t) can be written as
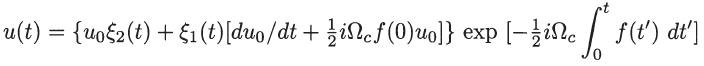
where u0 and du0/dt represent the initial position and velocity, respectively.
Considering now that the particle is initially (t = 0) at the origin and moving with velocity v0 along the negative y axis, that is, u0 = 0 and du0/dt = –iv0, show that
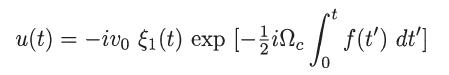
and, consequently,
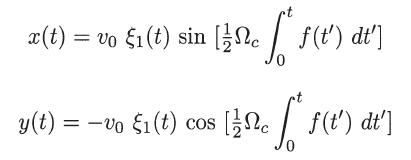
Step by Step Answer:






