From the expression for m , obtained in part (a) of problem 20.4, verify that for

From the expression for σ_m, obtained in part (a) of problem 20.4, verify that for p = 2 the momentum transfer cross section is given by

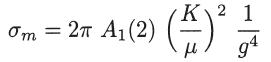
where A₁(2) is given by (with ℓ = 1 and p = 2)

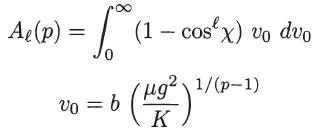
Consequently, the velocity-dependent collision frequency, defined by

varies as g^–3 . This inverse dependence on g accounts for the so-called electron runaway effect. This implies that in the presence of a sufficiently large electric field E, some electrons will gain enough kinetic energy between collisions so as to decrease their cross section and the collision frequency, which in turn allows them to pick up more energy from the field and decrease even further their cross section and collision frequency. If E is large enough, the collision frequency may fall so fast that these electrons will form an accelerated beam of runaway electrons.

Data from Problem 20.4(a).

Consider a general inverse-power interparticle force of the form

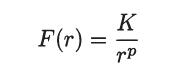
where K is a constant and p is a positive integer number.

(a) Determine expressions for the scattering angle χ, for the differential scattering cross section σ(χ, ∈), for the total scattering cross section at, and for the momentum transfer cross section σ_m.

Transcribed Image Text:

A1(2) (1) н 21 om = 2п A1(2)