Critical Radius of a Spherical Quantum Dot with Finite Barrier Height Assume that a quantum dot has a spherical shape with radius R and is surrounded by a medium of higher bandgap such as AlGaAs. The potential barrier at the conduction band is ΔEc at all points in the surface of the sphere. The potential well is a square well of height, ΔE_c, for r > R and is 0 for r < R. Let us consider the simplest case of zero angular momentum (l = 0), and then it follows that the wavefunctions Ψ (r^→) depends only on the radial part. When l ¼ 0 and Ψ(r^→)= R(r) = ϕ (r)=r, Eq. (15.56) reduces to:

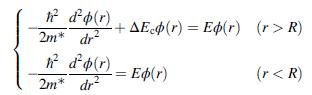
The solution of the above equation is the same as the one with a one-dimensional finite potential well. Find the critical radius below which there is no bound state of one electron in the quantum dot.

Transcribed Image Text:

* d'p+ AE(r) = E¢(r) (r>R) 2m* dr? d(r) 2= E¢(r) (r< R) 2m* dr?