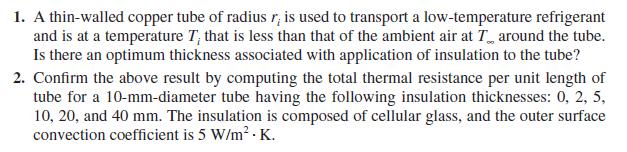
The concept of a critical insulation radius was introduced in Example 3.6. Consider the thin-walled copper tube and insulation of the example. Now, the tube temperature is \(-10^{\circ} \mathrm{C}\) and it is suspended horizontally in quiescent air at \(25^{\circ} \mathrm{C}\). Neglecting radiation, determine the heat transfer rate per unit tube length for an insulation thickness of \(10 \mathrm{~mm}\). Graph the conduction, convection, and total thermal resistances for a unit length for insulation thickness \(r-r_{i}\) over the range \(0 \leq r-r_{i} \leq\) \(50 \mathrm{~mm}\), and compare your results to that of the example. Repeat the calculation and the graphing exercise for a tube diameter of \(1 \mathrm{~mm}\). For each case, does a critical insulation radius exist? Why or why not? Evaluate air properties at \(285 \mathrm{~K}\).

Data From Example 3.6:-

Transcribed Image Text:

The possible existence of an optimum insulation thickness for radial systems is suggested by the presence of competing effects associated with an increase in this thickness. In par- ticular, although the conduction resistance increases with the addition of insulation, the convection resistance decreases due to increasing outer surface area. Hence there may exist an insulation thickness that minimizes heat loss by maximizing the total resistance to heat transfer. Resolve this issue by considering the following system.