In 1938 Dr. Frank Benford, a physicist at General Electric, noticed that, given a list of numbers, more numbers started with the digit 1 than any other digit. He analyzed 20,222 sets of numbers including areas of rivers, baseball statistics, numbers in magazine articles, and street addresses. He concluded that the probability of the numeral d appearing as the first digit log(1 + 1/d) and this property is known as Benford’s Law. The probabilities are shown in the table in Exercise 11.4.20. 

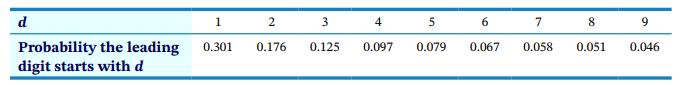
a. According to Benford’s Law, what is the probability that the leading digit of a randomly chosen number is 1 or 2? 

b. According to Benford’s Law, what is the probability that the leading digit of a randomly chosen number is more than 2? 

c. What is the expected value of the leading digit of randomly chosen numbers according to Benford’s Law? 

d. What is the standard deviation of the leading digit of randomly chosen numbers according to Benford’s Law?

Transcribed Image Text:

d Probability the leading digit starts with d 1 0.301 2 0.176 3 0.125 4 0.097 5 0.079 6 0.067 7 8 0.058 0.051 9 0.046