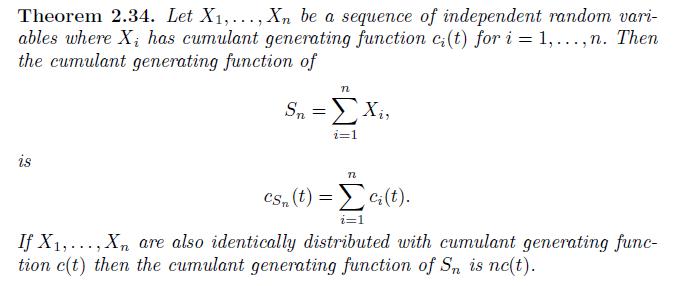
Prove Theorem 2.34. That is, suppose that \(X_{1}, \ldots, X_{n}\) be a sequence of independent random variables where \(X_{i}\) has cumulant generating function \(c_{i}(t)\) for \(i=1, \ldots, n\). Then prove that the cumulant generating function of

\[S_{n}=\sum_{i=1}^{n} X_{i}\]

is

\[c_{S_{n}}(t)=\sum_{i=1}^{n} c_{i}(t)\]

Transcribed Image Text:

Theorem 2.34. Let X1,..., Xn be a sequence of independent random vari- ables where X; has cumulant generating function c(t) for i = 1,...,n. Then the cumulant generating function of n Sn = Xi, i=1 is n cs, (t) = c(t). i=1 If X1, Xn are also identically distributed with cumulant generating func- tion c(t) then the cumulant generating function of Sn is nc(t).