There were 79 countries who competed in the 1996 Olympics and won at least one medal. For each of these countries, let MEDALS be the total number of medals won, POPM be population in millions, and GDPB be GDP in billions of 1995 dollars. Using these data we estimate the regression model \(M E D A L S=\beta_{1}+\beta_{2} P O P M+\beta_{3} G D P B+e\) to obtain

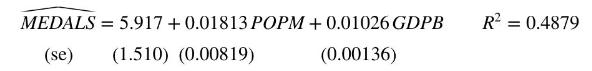
a. Given assumptions MR1-MR6 hold, interpret the coefficient estimates for \(\beta_{2}\) and \(\beta_{3}\).

b. Interpret \(R^{2}\).

c. Using a \(1 \%\) significance level, test the hypothesis that there is no relationship between the number of medals won and GDP against the alternative that there is a positive relationship. What happens if you change the significance level to \(5 \%\) ?

d. Using a \(1 \%\) significance level, test the hypothesis that there is no relationship between the number of medals won and population against the alternative that there is a positive relationship. What happens if you change the significance level to \(5 \%\) ?

e. Test the following hypotheses using a \(5 \%\) significance level:
i. \(H_{0}: \beta_{2}=0.01\) against the alternative \(H_{1}: \beta_{2} eq 0.01\)
ii. \(H_{0}: \beta_{2}=0.02\) against the alternative \(H_{1}: \beta_{2} eq 0.02\)
iii. \(H_{0}: \beta_{2}=0.03\) against the alternative \(H_{1}: \beta_{2} eq 0.03\)
iv. \(H_{0}: \beta_{2}=0.04\) against the alternative \(H_{1}: \beta_{2} eq 0.04\). Are these test results contradictory? Why or why not?

f. Find a \(95 \%\) interval estimate for \(\beta_{2}\) and comment on it.

Transcribed Image Text:

MEDALS 5.917 +0.01813 POPM +0.01026 GDPB = (se) (1.510) (0.00819) (0.00136) R = 0.4879