Let \((X, \mathscr{A}, \mu)\) be a measure space and \((X, \overline{\mathscr{A}}, \bar{\mu})\) its completion (see Problem 4.15 ). Show that a function \(\phi: X ightarrow \mathbb{R}\) is \(\overline{\mathscr{A}} / \mathscr{B}(\mathbb{R})\)-measurable if, and only if, there are \(\mathscr{A} / \mathscr{B}(\mathbb{R})\) measurable functions \(f, g: X ightarrow \mathbb{R}\) such that \(f \leqslant \phi \leqslant g\) and \(\mu\{f eq g\}=0\).

[ use simple functions and the sombrero lemma.]

Data from problem 4.15

Transcribed Image Text:

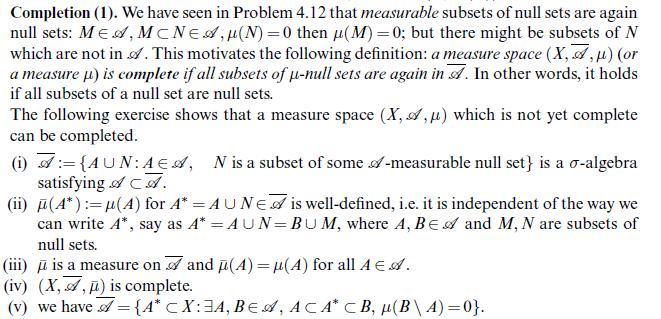
Completion (1). We have seen in Problem 4.12 that measurable subsets of null sets are again null sets: MEA, MCNEA, (N)=0 then (M)= 0; but there might be subsets of N which are not in. This motivates the following definition: a measure space (X, A, ) (or a measure ) is complete if all subsets of u-null sets are again in A. In other words, it holds if all subsets of a null set are null sets. The following exercise shows that a measure space (X, ,) which is not yet complete can be completed. (1) A:= {AUN: AA, N is a subset of some -measurable null set} is a -algebra satisfying CA. (ii) (4*):= (4) for A* = AUNE is well-defined, i.e. it is independent of the way we can write 4*, say as A* = AUN=BUM, where A, BEA and M, N are subsets of null sets. (iii) is a measure on and (4) = (A) for all A = A. (iv) (X, A, ) is complete. (v) we have = {A* CX: 3A, BEA, ACA* CB, (B\ 4)=0}.