State and prove Theorem 28.6 and Corollary 28.8 for an arbitrary compact interval ([a, b]). Data from
Question:
State and prove Theorem 28.6 and Corollary 28.8 for an arbitrary compact interval \([a, b]\).
Data from theorem 28.6
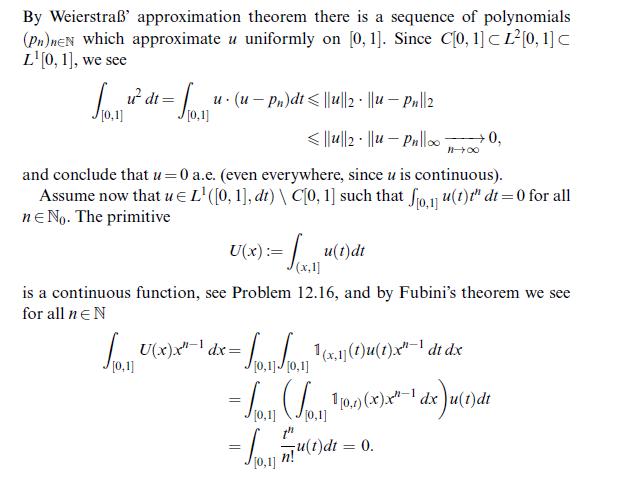
![Theorem 28.6 (Weierstra) Polynomials are dense in C[0, 1] w.r.t. uniform convergence. Proof (S. N. Bernstein)](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1705/9/8/7/29665af4ce02bec11705987295385.jpg)
![since the function p-p(1-p) attains its maximum at p=1/2. As u C[0, 1] is uniformly continuous, u(x) - u(y) |](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1705/9/8/7/30865af4cec958951705987308031.jpg)
Data from corollary 28.8
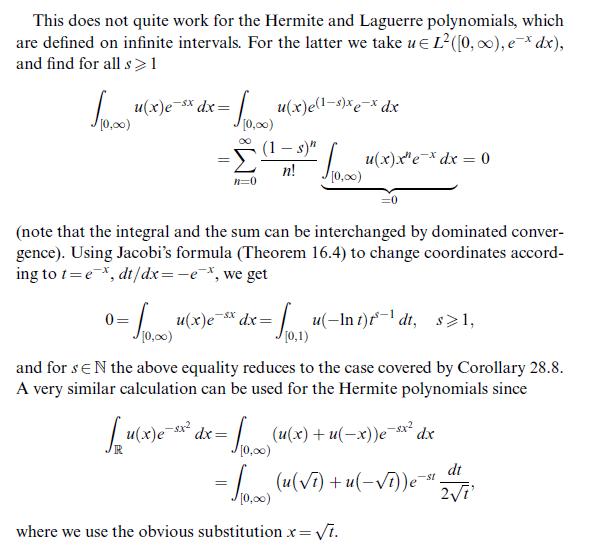
![Corollary 28.8 The monomials (t")neN, are complete in L= L ([0, 1], dt), that is, 0,11u(t)t" dt=0 for all](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1705/9/8/7/33865af4d0a60c5f1705987337820.jpg)
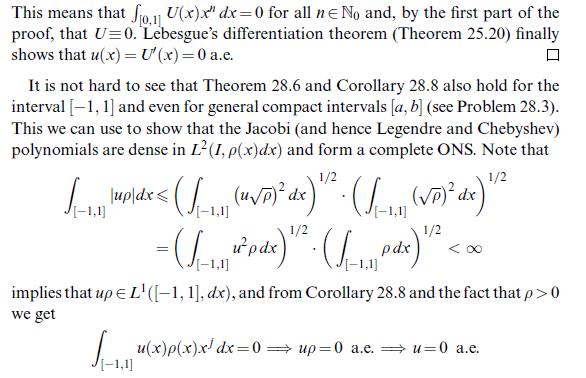
Transcribed Image Text:
Theorem 28.6 (Weierstra) Polynomials are dense in C[0, 1] w.r.t. uniform convergence. Proof (S. N. Bernstein) Take sequence (Si)ieN of independent2 measur- able functions on ([0, 1], [0, 1], dx) which are Bernoulli (p, 1 - p)-distributed, 0
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answer rating: 75% (4 reviews)
Theorem 286 The polynomials are dense in Ca b with respect to uniform convergence ...View the full answer

Answered By

Khurram shahzad
I am an experienced tutor and have more than 7 years’ experience in the field of tutoring. My areas of expertise are Technology, statistics tasks I also tutor in Social Sciences, Humanities, Marketing, Project Management, Geology, Earth Sciences, Life Sciences, Computer Sciences, Physics, Psychology, Law Engineering, Media Studies, IR and many others.
I have been writing blogs, Tech news article, and listicles for American and UK based websites.
4.90+
5+ Reviews
17+ Question Solved
Related Book For 

Question Posted:
Students also viewed these Business questions
-
A compare-exchange operation on two array elements A[i] and A[j], where i < j, has the form COMPARE-EXCHANGE (A, i, j) 1 If A[i] > A[j] 2 exchange A[i] with A[j] After the compare-exchange operation,...
-
The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-5. Ivan's grandfather died and left a portfolio of municipal bonds. In 2012, they pay Ivan...
-
The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-6. On December 12, Irene purchased the building where her store is located. She paid...
-
In your own words, define or explain the terms or symbols (a) (b) [ ]; (c) Spectator ion; (d) Weak acid.
-
How accurately can the position of a 3.00-keV electron be measured assuming its energy is known to 1.00%?
-
Copper has compounds with copper(I) ion or copper(II) ion. A compound of copper and chlorine was treated with a solution of silver nitrate, AgNO3, to convert the chloride ion in the compound to a...
-
Consider the model: E1y2 = b0 + b1x1 + b2x2 + b3x3 + e where x1 is a quantitative variable and x2 and x3 are dummy variables describing a qualitative variable at three levels using the coding scheme...
-
Balance sheets for P Company and S Company on August 1, 2011, are as follows: Required: Prepare a workpaper for a consolidated balance sheet for P Company and its subsidiary on August 1, 2011, taking...
-
The following balance sheet for the Los Gatos Corporation was prepared by a recently hired accountant. In reviewing the statement you notice several errors. LOS GATOS CORPORATION Balance Sheet At...
-
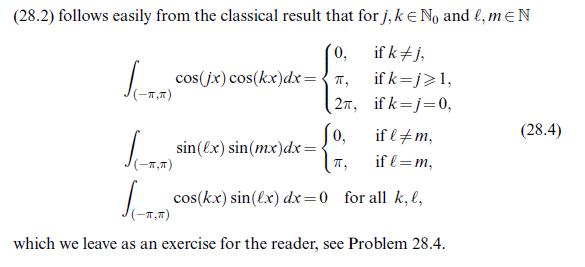
Prove the orthogonality relations (28.4) for the trigonometric system. Equation 28.4 (-T,T) cos(jx) cos(kx) dx = (-,) L sin (lx) sin(mx) dx = (-TT) 0, , 2, if lm, T, if l=m, cos(kx) sin(x) dx=0 for...
-
Use the Gram-Schmidt orthonormalization procedure to verify the formulae for the Chebyshev, Legendre, Laguerre and Hermite polynomials given in items 28.1 -28.5.
-
Separated by the Atlantic. Separated by more than 3,000 nautical miles and five time zones, money and foreign exchange markets in both London and New York are very efficient. The following...
-
Molina Company produces three products: A130, B324, and C587 All three products use the same direct material, Brac Unt data for the three products are in the provided table. (Click to view the unit...
-
MFGE 437 S21 - Homework 1 Submissions will be Online! Please scan your HWs and upload on Canvas Problem 1: A vertical milling machine is to be retrofitted with three identical DC servo motors. The...
-
On January 1, Palisades, Inc., acquired 100 percent of Sherwood Company's common stock for a fair value of $120,340,000 in cash and stock. The carrying amounts of Sherwood's assets and liabilities...
-
(1) A test balloon has an accelerometer attached to it. After you release it and start collecting data it is 5 ft in front of you and 16 ft above you, and it is moving 5 ft/s to your left and 4 ft/s...
-
484 ... Age of Accounts as of June 30, 2019 1-30 31-60 61-90 Over 90 Customer Name Days Days Days Days Total Balance Canyon Youth Club $ 250 $ 250 Crazy Tees 200 $ 150 350 Early Start Daycare $500...
-
Ethyl alcohol (C2H5OH(g)) at 25C is burned in a steady-flow adiabatic combustion chamber with 40 percent excess air that also enters at 25C. Determine the adiabatic flame temperature of the products...
-
The tractor is used to lift the 150-kg load B with the 24-mlong rope, boom, and pulley system. If the tractor travels to the right at a constant speed of 4 m/s, determine the tension in the rope when...
-
What is the difference between a value-added activity and a non-value-added activity? Provide two examples of non-value-added activities for each of the following: a. Fast-food restaurant b. Clothing...
-
How did activity-based costing help BuyGasCo Corporation settle its predatory pricing case?
-
What did the survey of 296 users of ABC and ABM show were the top two objectives in using these systems?
-
Each week you must submit an annotated bibliography. Entries of current events relating to the economic concepts and the impact on the company or the industry of your company. You must use acceptable...
-
Fluffy Toys Ltd produces stuffed toys and provided you with the following information for the month ended August 2020 Opening WIP Units 5,393 units Units Started and Completed 24,731 units Closing...
-
Part A Equipment 1,035,328 is incorrect Installation 44,672 is incorrect Anything boxed in red is incorrect sents 043/1 Question 9 View Policies Show Attempt History Current Attempt in Progress...

Study smarter with the SolutionInn App


