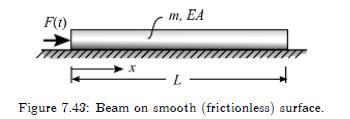
An unrestrained beam lies on a horizontal and smooth surface and at \(t=0\) is forced at one end by \(F(t)\), as shown in Figure 7.43. Derive the general response for any forcing function using the known eigenvalues and eigenfunctions. Then evaluate the specific steady-state response for the following specific forcing functions:

(a) \(F(t)=F_{0} u(t)\), where \(u(t)\) is the unit step function

(b) \(F(t)=\sin \Omega t\), for the case where \(\Omega=\omega_{n} / 2\)

(c) \(F(t)=1-t / t_{0}, 0 \leq t \leq t_{0}\)

(d) \(F(t)=\delta(t)\), where \(\delta(t)\) is the Dirac delta function.

Transcribed Image Text:

F(t) x m. EA Figure 7.43: Beam on smooth (frictionless) surface.