Let f () be an arbitrary scalar function of the scalar variable . We have learned that f (z ct) is a traveling-wave solution

Let f (ξ) be an arbitrary scalar function of the scalar variable ξ. We have learned that f (z − ct) is a traveling-wave solution of the one dimensional wave equation. In other words,

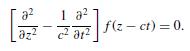
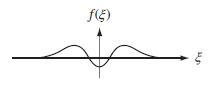
We have also learned that solutions of this equation can be localized, i.e, f (ξ ) can go to zero outside a finite interval of ξ . Now let ψ(x, y, z − ct) be a solution of the three-dimensional wave equation. Use the

information just given (and your knowledge of electrostatics) to prove that ψ cannot be localized in the x, y, and z directions simultaneously.

1 [2-237]f(2-ct) = 0.