The figure below shows a sample of “artificial matter” composed of infinite, parallel, filamentary wires. Each row of wires carries current in the opposite direction from the rows just above and below it. Each row is also displaced (vertically and horizontally) by a distance a/2 from the rows just above and below it. Each wire feels a restoring force −ku (per unit length) if it moves a distance u (perpendicular to its length) from its equilibrium position shown in the diagram. Each wire (mass per unit length m) also feels a damping force −mγ u̇  when it is in motion.

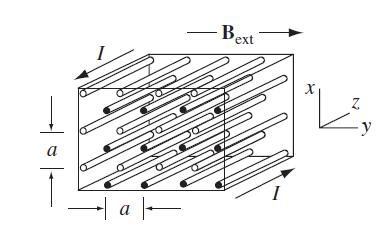
(a) Explain why the magnetic force exerted on each wire by the other wires can be neglected (compared to the force from an external magnetic field) when u is small.

(b) Let +z be the direction of positive current I . Show that a uniform magnetic field B = ŷB exp(−iωt) inside the sample induces a steady-state displacement of each wire in the x-direction by an amount

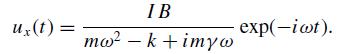
(c) The displacements in part (a) expose current sheets at the top and bottom faces (parallel to the y-z plane) of the sample. Taking this into account, show that, when exposed to an external field B_ext = ŷ B_ext exp(−iωt), the total field inside the sample satisfies the self-consistency equation

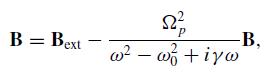
where ω²₀ = k/m and Ω²_p = 2μ₀ I²/ma².
(d) Use the matching conditions for H to derive an expression for B/Bext. Combine this information with the formula derived in part (c) to obtain an expression for the magnetic permeability μ(ω) of the “wire matter” sample.

Transcribed Image Text:

a a l -Bext X