Let () / 0 = 1 2 p / 2 be the dielectric function

Let ϵ(ω) / ϵ₀ = 1 − ω²_p /ω² be the dielectric function of a plasma where ωp is the plasma frequency. In a typical laboratory or astrophysical environment, any attempt to create a voltage drop V (t) = V cos ωt across the plasma generates a region of vacuum (called the “sheath”) on either side of the plasma volume as indicated in the one-dimensional sketch below.

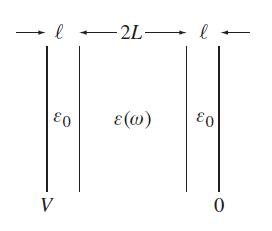
(a) Derive expressions for the uniform electric field E_P (t) = E_P cos ωt in the plasma and for the uniform electric field E_S (t) = E_S cos ωt in the sheath. Assume there is no free charge anywhere. Assume also that ω_p is small enough that an electrostatic approximation is always valid.
(b) Plot the field amplitudes E_P and E_S on the same graph as a function of frequency. Discuss where the voltage drop occurs and why when (1) ω p, (2) ω = ω_p, and (3) ω >> ω_p.
(c) Make an “LC circuit” interpretation of the resonance behavior at ω = ωp / √ 1 + L/ℓ.

Transcribed Image Text:

-2L- 80 ε(w) V l 80 0